Essential Division Worksheets for Third Graders: Master the Basics

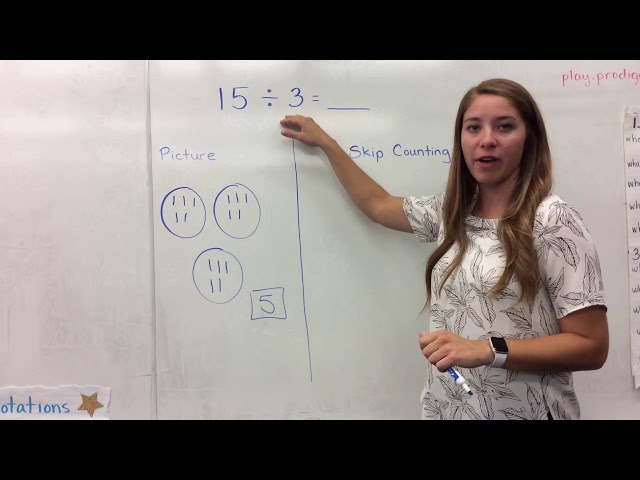
Division for Third Graders

Division is a fundamental mathematical operation that involves dividing a whole into equal parts. For third graders, understanding division can be challenging, but using charts and diagrams can make the concept more accessible and engaging.

To create effective division charts and diagrams, follow these steps:

1. Identify the dividend, divisor, and quotient: The dividend is the number being divided, the divisor is the number dividing the dividend, and the quotient is the answer.
2. Create a visual representation: Draw a rectangle or circle to represent the dividend. Divide the shape into equal parts to represent the divisor.
3. Count the groups: Count the number of groups created by the division. This represents the quotient.
4. Label the chart or diagram: Clearly label the dividend, divisor, and quotient on the chart or diagram.

Here are some examples of division charts and diagrams:

• Division Rectangle: Divide a rectangle into equal parts to represent the divisor. Count the number of groups to find the quotient.
• Division Circle: Divide a circle into equal parts to represent the divisor. Count the number of groups to find the quotient.
• Array Diagram: Arrange objects into rows and columns to create an array. The number of rows represents the divisor, and the number of columns represents the quotient.

Using charts and diagrams to teach division offers several benefits:

• Visual aids make abstract concepts more concrete.
• Charts and diagrams provide a hands-on approach to learning.
• They help students develop problem-solving and critical thinking skills.

In conclusion, charts and diagrams are valuable tools for teaching division to third graders. By following the steps outlined above, you can create effective visual aids that will help students understand and apply this important mathematical operation.

Essential Aspects of Division for Third Graders

Division is a fundamental mathematical operation that involves dividing a whole into equal parts. For third graders, understanding division can be challenging, but focusing on the following six key aspects can make the concept more accessible:

• Dividend: The number being divided.
• Divisor: The number dividing the dividend.
• Quotient: The answer to a division problem.
• Equal Groups: Division involves dividing a whole into equal groups.
• Repeated Subtraction: Division can be thought of as repeated subtraction.
• Arrays: Arrays can be used to represent division problems visually.

These aspects are interconnected and essential for understanding division. For example, the dividend represents the whole that is being divided, the divisor represents the size of each equal group, and the quotient represents the number of equal groups. Repeated subtraction and arrays provide alternative ways to think about and solve division problems.

By focusing on these key aspects, third graders can develop a solid understanding of division and its applications in everyday life.

Dividend

In the context of division for third graders, the dividend is the number being shared or divided into equal parts. Understanding the concept of the dividend is crucial for students to grasp the fundamentals of division.

• Facet 1: Identifying the Dividend
  In a division problem, the dividend is the starting number that is to be divided. For instance, in the problem 12 3 = 4, the dividend is 12.
• Facet 2: Relating the Dividend to the Whole
  The dividend represents the whole quantity that is being divided into smaller, equal parts. In the example above, the dividend of 12 represents the total number of items that need to be shared equally.
• Facet 3: Connecting the Dividend to the Quotient
  The quotient in a division problem represents the number of equal groups or parts that result from dividing the dividend by the divisor. The dividend, divisor, and quotient are interconnected, and understanding their relationship is essential for solving division problems.
• Facet 4: Visualizing the Dividend using Arrays
  Arrays can be used to visualize the dividend and the division process. For example, an array of 12 objects arranged in 3 rows and 4 columns represents a dividend of 12 and a divisor of 3. The rows in the array represent the divisor, and the columns represent the quotient.

By exploring these facets of the dividend, third graders can develop a comprehensive understanding of its role in division and apply this knowledge to solve division problems effectively.

Divisor

In the realm of division for third graders, the divisor plays a pivotal role, acting as the number that divides the dividend, the total quantity being shared. Understanding the concept of the divisor is paramount for students to grasp the mechanics and applications of division.

The divisor dictates the size and number of equal parts into which the dividend will be divided. For instance, in the problem 12 3 = 4, the divisor 3 determines that the dividend 12 will be divided into 3 equal groups. The divisor, therefore, directly influences the quotient, the result of the division, as it determines how many equal parts the dividend will be split into.

Introducing the concept of the divisor to third graders can be facilitated through hands-on activities and visual aids. Using objects such as blocks or counters, students can physically divide a set into equal groups, demonstrating the role of the divisor in determining the number and size of these groups. Additionally, arrays, mathematical representations using rows and columns, can be employed to illustrate the divisor as the number of rows, visually depicting how the dividend is partitioned into equal parts.

Comprehending the divisor’s role is not only crucial for solving division problems but also lays the foundation for understanding more complex mathematical concepts, such as fractions and ratios. By grasping the concept of the divisor, third graders develop a strong foundation in division, a skill that will serve them well in their mathematical journey and beyond.

Quotient

In the realm of division for third graders, the quotient holds a central place as the answer to the division problem. Understanding the quotient is crucial for students to grasp the essence and applications of division.

• Facet 1: Defining the Quotient
  The quotient represents the number of equal groups or parts that result from dividing the dividend by the divisor. In the problem 12 3 = 4, the quotient 4 indicates that the dividend 12 has been divided into 4 equal groups.
• Facet 2: Relating the Quotient to the Dividend and Divisor
  The quotient is directly related to both the dividend and the divisor. A larger dividend will generally result in a larger quotient, while a larger divisor will generally result in a smaller quotient.
• Facet 3: Visualizing the Quotient using Arrays
  Arrays can be used to visualize the quotient. In the problem 12 3 = 4, an array of 12 objects arranged in 3 rows and 4 columns would represent the quotient 4.
• Facet 4: Applying the Quotient in Real-Life Situations
  The quotient finds practical applications in everyday life. For instance, if a pizza is cut into 8 slices and 4 people share it equally, the quotient 2 represents the number of slices each person receives.

By exploring these facets of the quotient, third graders develop a comprehensive understanding of its role in division and apply this knowledge to solve division problems and make sense of real-world scenarios.

Equal Groups

In the realm of division for third graders, the concept of equal groups holds paramount importance as a foundational pillar upon which the understanding of division rests. Division, in essence, revolves around the notion of partitioning a wholethe dividendinto equal parts or groups, determined by the divisor. This concept of equal groups is pivotal for third graders to grasp, as it forms the very basis of division and its applications.

Consider a real-life example: Imagine a pizza cut into 8 equal slices. If 4 friends share this pizza equally, each friend receives 2 slices. Here, the pizza represents the dividend, the number of slices (8) represents the divisor, and the number of slices each friend receives (2) represents the quotient. This example illustrates how division involves dividing a whole (the pizza) into equal groups (the slices) to determine the equal share (the quotient).

Understanding equal groups in division empowers third graders to solve real-world problems and develop a deeper comprehension of mathematical concepts. It enables them to visualize the division process and make connections between the dividend, divisor, and quotient. Furthermore, this understanding serves as a stepping stone for more complex mathematical operations, such as fractions and ratios.

In conclusion, the concept of equal groups is an indispensable component of division for third graders. By grasping this concept, students lay a solid foundation for their mathematical journey and develop essential problem-solving skills that extend beyond the classroom.

Repeated Subtraction

In the realm of division for third graders, the concept of repeated subtraction offers a powerful lens through which to understand this fundamental mathematical operation. Repeated subtraction provides a relatable and intuitive approach to division, making it more accessible and meaningful for young learners.

• Facet 1: Repeated Subtraction as a Foundation
  Division can be thought of as the repeated subtraction of the divisor from the dividend. For instance, in the problem 12 3, we can solve it by subtracting 3 from 12 repeatedly: 12 – 3 = 9, 9 – 3 = 6, 6 – 3 = 3. This process reveals the quotient, which is 4, indicating that 12 can be divided into 3 equal groups of 4.
• Facet 2: Connecting Repeated Subtraction to Arrays
  Arrays provide a visual representation of repeated subtraction in division. Consider an array with 12 objects arranged in 3 rows of 4 columns. Subtracting 3 objects (the divisor) repeatedly from the first row, then the second row, and finally the third row, demonstrates the division process and reveals the quotient of 4.
• Facet 3: Applying Repeated Subtraction in Real-Life Situations
  Repeated subtraction finds practical applications in everyday life. For example, if a farmer has 12 apples and wants to distribute them equally among 3 baskets, he can use repeated subtraction to determine how many apples to put in each basket. By subtracting 3 apples at a time, he will find that each basket receives 4 apples.
• Facet 4: Building Fluency through Repeated Subtraction
  Repeated subtraction can enhance students’ fluency in division. By repeatedly subtracting the divisor from the dividend until the remainder is less than the divisor, students develop a strong understanding of the division process and improve their problem-solving skills.

In conclusion, the concept of repeated subtraction provides a valuable framework for understanding division for third graders. Through its intuitive approach, visual representations, and real-life applications, repeated subtraction helps students grasp the essence of division and develop essential mathematical skills.

Arrays

Arrays, two-dimensional arrangements of elements in rows and columns, serve as powerful visual aids in representing division problems, making them more accessible and understandable for third graders.

Arrays provide a concrete representation of the division process. By arranging objects or symbols in rows and columns, students can visualize the dividend as the total number of objects and the divisor as the number of objects in each row. The number of columns in the array represents the quotient, indicating the number of equal groups that can be formed.

For example, consider a division problem: 12 3. We can represent this problem using an array of 12 objects arranged in 3 rows. Each row contains 4 objects, representing the divisor of 3. The number of rows, which is 4, represents the quotient, indicating that 12 can be divided into 3 equal groups of 4.

Arrays not only aid in understanding the division concept but also facilitate problem-solving. By manipulating the objects or symbols in the array, students can physically demonstrate the division process and find the quotient. This hands-on approach enhances their understanding and retention.

Moreover, arrays provide a visual representation that aligns with the concept of repeated subtraction, another method for solving division problems. Each row in the array can be thought of as one subtraction of the divisor from the dividend, making the connection between the two methods more apparent.

In conclusion, arrays play a vital role in teaching division for third graders. They provide a visual representation of the division process, making it more accessible and understandable. Arrays facilitate problem-solving, enhance understanding, and connect division to the concept of repeated subtraction.

Division, a fundamental mathematical operation, is a crucial skill for third graders to master. It involves dividing a set (the dividend) into equal-sized groups (the divisors), resulting in the number of groups (the quotient).

Understanding division empowers students to solve real-world problems and develop higher-order thinking skills. It strengthens their number sense, reasoning abilities, and problem-solving strategies. Historically, division has been an essential tool for trade, commerce, and scientific advancements.

To fully grasp division, third graders explore various concepts:

Frequently Asked Questions about Division for Third Graders

Division, a key mathematical operation, plays a vital role in problem-solving and critical thinking. Here are answers to some common questions about division for third graders:

Question 1: What is division?

Answer: Division is the process of sharing a set (the dividend) into equal-sized groups (the divisors), resulting in the number of groups (the quotient).

Question 2: Why is division important?

Answer: Division helps us solve everyday problems, such as sharing objects fairly, measuring ingredients for recipes, and understanding fractions.

Question 3: How can I help my child understand division?

Answer: Use hands-on activities like sharing objects into groups, drawing arrays, and using number lines. Connect division to real-life situations to make it more meaningful.

Question 4: What are some common misconceptions about division?

Answer: Some misconceptions include thinking that division is only about subtracting or that the divisor can be zero. It’s important to address these misconceptions and provide clear explanations.

Question 5: What are some tips for solving division problems?

Answer: Encourage your child to use strategies like skip-counting, repeated subtraction, and estimation. Also, practice division facts to improve fluency.

Question 6: What resources are available to help third graders with division?

Answer: Utilize online games, interactive websites, and printable worksheets to supplement classroom learning and provide additional practice.

Division is a foundational mathematical concept that empowers third graders to solve problems and develop their reasoning skills. By addressing common questions and providing effective strategies, we can support their understanding of this important operation.

Continue to the next section for more in-depth information on division for third graders.

Conclusion

Division, a crucial mathematical operation, empowers third graders to solve problems and develop their reasoning skills. This article explored key concepts, strategies, and misconceptions related to division, providing a comprehensive understanding for educators and parents.

Understanding division is not only about mastering computational skills but also about developing critical thinking and problem-solving abilities. By fostering a strong foundation in division, we equip third graders with essential tools for future mathematical success and everyday life applications.

Youtube Video: