Master Fraction Arithmetic: Adding and Subtracting with Unlike Denominators Worksheet

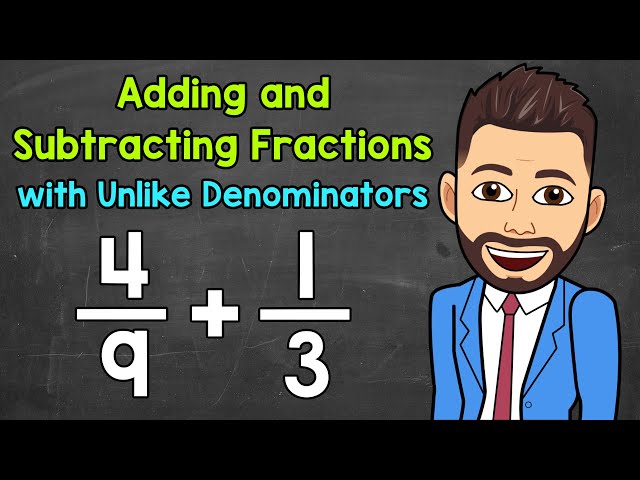
Chart and Diagram Examples of Adding and Subtracting Fractions with Unlike Denominators

Adding and subtracting fractions with unlike denominators can be a challenging concept for students to grasp. Using charts and diagrams can help make the process more visual and easier to understand.

Here are some examples of charts and diagrams that can be used to teach adding and subtracting fractions with unlike denominators:

• Fraction circles: Fraction circles are a great way to visualize fractions and see how they compare to each other. To create a fraction circle, divide a circle into equal parts and shade in the parts that represent the fraction. For example, to create a fraction circle for 1/2, divide the circle into two equal parts and shade in one of the parts.
• Fraction strips: Fraction strips are another helpful visual aid for teaching fractions. Fraction strips are long strips of paper that are divided into equal parts. Each part represents a fraction of the whole. For example, a fraction strip that is divided into 10 equal parts could be used to represent the fraction 1/10.
• Number lines: Number lines can be used to add and subtract fractions. To add fractions on a number line, start at the zero point and move to the right by the numerator of the first fraction. Then, move to the right again by the numerator of the second fraction. The final point represents the sum of the two fractions.

Benefits of using charts and diagrams to teach adding and subtracting fractions with unlike denominators:

• Charts and diagrams can help make the process more visual and easier to understand.
• Charts and diagrams can help students see how fractions compare to each other.
• Charts and diagrams can help students develop a deeper understanding of fractions.

Conclusion: Using charts and diagrams is a great way to teach adding and subtracting fractions with unlike denominators. Charts and diagrams can help make the process more visual and easier to understand, and they can help students develop a deeper understanding of fractions.

Key Aspects of Adding and Subtracting Fractions with Unlike Denominators

Adding and subtracting fractions with unlike denominators is a fundamental skill in mathematics. Understanding the key aspects of this topic is essential for students to develop a strong foundation in fractions.

• Finding a common denominator: The first step in adding or subtracting fractions with unlike denominators is to find a common denominator. This means finding a number that is divisible by both denominators.
• Equivalent fractions: Equivalent fractions are fractions that have the same value, even though they may look different. To add or subtract fractions with unlike denominators, you can convert them to equivalent fractions with a common denominator.
• Adding the numerators: Once you have found a common denominator, you can add or subtract the numerators of the fractions. The denominator remains the same.
• Simplifying the fraction: After adding or subtracting the numerators, you may need to simplify the fraction. This means reducing the fraction to its lowest terms.
• Borrowing: In some cases, you may need to borrow from the whole number when adding or subtracting fractions. This is similar to the process of borrowing in subtraction.
• Visual models: Visual models, such as fraction circles or fraction strips, can be helpful for understanding the concepts of adding and subtracting fractions with unlike denominators.
• Real-world applications: Adding and subtracting fractions with unlike denominators is a skill that is used in many real-world applications, such as cooking, carpentry, and engineering.

These key aspects provide a comprehensive overview of the topic of adding and subtracting fractions with unlike denominators. By understanding these aspects, students can develop a strong foundation in this essential mathematical skill.

Finding a common denominator

Finding a common denominator is a crucial step in adding and subtracting fractions with unlike denominators. The common denominator serves as the common unit of measurement for the fractions, allowing them to be combined or compared. Without a common denominator, it is not possible to perform these operations accurately.

To find a common denominator, we can use the following methods:

• Prime factorization: Prime factorization involves breaking down each denominator into its prime factors. The common denominator is then found by multiplying the highest power of each prime factor that appears in any of the denominators.
• Least common multiple (LCM): The LCM of two or more numbers is the smallest number that is divisible by all of the numbers. To find the LCM of the denominators, we can list the multiples of each denominator and find the smallest number that appears in all of the lists.

Once we have found a common denominator, we can convert each fraction to an equivalent fraction with the common denominator. This is done by multiplying both the numerator and denominator of each fraction by the same number. For example, to convert the fraction 1/2 to an equivalent fraction with a denominator of 6, we would multiply both the numerator and denominator by 3, resulting in the fraction 3/6.

Finding a common denominator is an essential skill for adding and subtracting fractions with unlike denominators. By understanding this concept, students can develop a strong foundation in fractions and perform these operations accurately and efficiently.

Equivalent fractions

Understanding equivalent fractions is crucial for adding and subtracting fractions with unlike denominators. Equivalent fractions provide a way to represent the same quantity using different combinations of numerators and denominators. This concept is fundamental to the process of finding a common denominator, a key step in adding and subtracting fractions with unlike denominators.

• Converting to equivalent fractions: Converting fractions to equivalent fractions with a common denominator allows for easy addition and subtraction. By multiplying both the numerator and denominator of a fraction by the same number, we can create an equivalent fraction with a different denominator while maintaining the same value.
• Simplifying fractions: Converting fractions to equivalent fractions also helps in simplifying fractions. By reducing fractions to their simplest form, we can work with smaller numbers and avoid unnecessary calculations.
• Real-world applications: Equivalent fractions have practical applications in various fields. For example, in cooking, scaling recipes requires converting fractions to equivalent fractions with a common denominator to adjust ingredient quantities accurately.

In the context of “adding and subtracting fractions with unlike denominators worksheet,” understanding equivalent fractions is essential for solving problems accurately. Worksheets often involve exercises where students need to convert fractions to equivalent fractions with a common denominator before performing addition or subtraction operations. By mastering this concept, students can develop a strong foundation in fraction operations and apply it effectively in various mathematical scenarios.

Adding the numerators

In the context of “adding and subtracting fractions with unlike denominators worksheet,” understanding the concept of adding the numerators is essential for solving problems accurately. Worksheets often involve exercises where students need to add or subtract fractions with unlike denominators, and the ability to add the numerators correctly is a crucial step in the process.

• Understanding the rule: The rule of adding the numerators, while keeping the denominator the same, provides a clear and systematic approach to combining fractions with unlike denominators. This rule helps students avoid common errors and ensures accurate calculations.
• Simplifying the process: Adding the numerators simplifies the process of adding fractions with unlike denominators. By focusing on the numerators and keeping the denominator the same, students can avoid dealing with complex calculations involving the denominators.
• Real-world applications: Adding fractions with unlike denominators is a skill that is used in various real-world applications. For example, in carpentry, adding fractions with unlike denominators is necessary to calculate measurements and ensure accurate cuts. Understanding this concept is essential for completing tasks efficiently and precisely.

Overall, the concept of adding the numerators is a fundamental aspect of “adding and subtracting fractions with unlike denominators worksheet.” By mastering this concept, students can develop a strong foundation in fraction operations and apply it effectively in various mathematical scenarios.

Simplifying the fraction

Simplifying fractions is an essential part of adding and subtracting fractions with unlike denominators. When we add or subtract the numerators of fractions with unlike denominators, the resulting fraction may not be in its simplest form. Simplifying the fraction means reducing it to its lowest terms, where the numerator and denominator have no common factors other than 1.

• Reducing to lowest terms: Simplifying a fraction involves reducing it to its lowest terms, where the numerator and denominator are as small as possible. This makes it easier to work with the fraction and compare it to other fractions.
• Finding common factors: To simplify a fraction, we need to find the common factors of the numerator and denominator. Common factors are numbers that divide evenly into both the numerator and denominator.
• Dividing by common factors: Once we have found the common factors, we can divide both the numerator and denominator by those factors. This reduces the fraction to its simplest form.
• Real-world applications: Simplifying fractions is a skill that is used in various real-world applications. For example, in cooking, simplifying fractions is necessary to scale recipes and ensure accurate measurements.

In the context of “adding and subtracting fractions with unlike denominators worksheet,” simplifying the fraction is an important step after adding or subtracting the numerators. By simplifying the fraction, students can reduce it to its lowest terms and make it easier to work with. This helps to ensure accurate calculations and a better understanding of fractions.

Borrowing

In the context of “adding and subtracting fractions with unlike denominators worksheet,” the concept of borrowing plays a significant role in certain scenarios. Borrowing is a technique used when the numerator of the fraction being added or subtracted is greater than the denominator. This process is similar to the concept of borrowing in subtraction, where we borrow from the next digit to the left.

• Understanding the concept of borrowing: Borrowing involves decomposing the whole number into smaller units, such as tenths or hundredths, to facilitate the borrowing process.
• Applying the borrowing technique: When adding fractions with unlike denominators, borrowing may be necessary to ensure that the fraction being added is less than the denominator. This allows for proper addition and reduces the risk of errors.
• Real-world applications: Borrowing is a crucial skill in various real-world applications, such as financial calculations, measurement conversions, and recipe adjustments. Understanding this concept is essential for accurate calculations and problem-solving.

In summary, borrowing is an important aspect of “adding and subtracting fractions with unlike denominators worksheet.” By understanding the concept of borrowing, students can effectively add and subtract fractions with unlike denominators, ensuring accurate calculations and a deeper comprehension of fractions.

Visual models

Visual models play a significant role in the context of “adding and subtracting fractions with unlike denominators worksheet” as they provide a hands-on and interactive approach to understanding the concepts involved. These models make it easier for students to visualize the process of adding and subtracting fractions with unlike denominators, promoting a deeper comprehension of the underlying principles.

• Concrete representation: Visual models provide a concrete representation of fractions, making them more tangible and easier to understand for students. Fraction circles and fraction strips allow students to see the relative sizes of fractions and how they can be combined or subtracted.
• Interactive manipulation: Visual models allow for interactive manipulation, enabling students to explore the concepts of adding and subtracting fractions with unlike denominators in a hands-on manner. By physically manipulating the models, students can gain a better understanding of how fractions interact.
• Real-world applications: Visual models can be linked to real-world applications, making the learning process more meaningful for students. For example, using fraction circles to represent pizzas or fraction strips to represent lengths of fabric helps students see the practical relevance of adding and subtracting fractions.
• Scaffolding for abstract concepts: Visual models serve as scaffolding for abstract concepts, providing a bridge between concrete and abstract thinking. They help students build a foundation of understanding before moving on to more abstract representations of fractions.

In summary, visual models are a valuable tool in “adding and subtracting fractions with unlike denominators worksheet” as they provide a concrete, interactive, and meaningful way to understand the concepts involved. By utilizing visual models, students can develop a deeper comprehension of fractions and their operations.

Real-world applications

Understanding how to add and subtract fractions with unlike denominators is not only crucial in academic settings but also has significant practical applications in various real-world scenarios. This concept is commonly encountered in fields such as cooking, carpentry, and engineering, where accurate calculations and measurements are essential.

• In cooking, recipes often involve precise measurements of ingredients, many of which are expressed in fractional form. Adding and subtracting fractions with unlike denominators allows cooks to adjust recipe quantities based on the number of servings or dietary restrictions, ensuring the correct proportions of ingredients for a successful dish.
• In carpentry, fractions are used to represent measurements for cutting and assembling materials. Carpenters need to be able to add and subtract fractions with unlike denominators to determine the total length of materials required, ensuring accurate cuts and proper fitting of components.
• In engineering, fractions are used in calculations related to forces, ratios, and proportions. Engineers need to be able to add and subtract fractions with unlike denominators to determine the correct values for these parameters, ensuring the safety and efficiency of engineered structures and systems.

Incorporating real-world applications into “adding and subtracting fractions with unlike denominators worksheet” provides students with a deeper understanding of the practical relevance of this mathematical concept. By connecting classroom learning to real-life scenarios, students can develop a stronger appreciation for the importance of fractions and their applications in various professions and everyday life.

A worksheet on adding and subtracting fractions with unlike denominators is an educational resource designed to provide practice and reinforce the mathematical concept of adding and subtracting fractions that have different denominators.

The importance of understanding how to add and subtract fractions with unlike denominators lies in its widespread applications in various fields and everyday life situations. It is a fundamental skill that enables individuals to solve problems involving fractional quantities accurately, which is crucial in areas like cooking, carpentry, engineering, and scientific calculations.

FAQs on Adding and Subtracting Fractions with Unlike Denominators

This section addresses frequently asked questions and clarifies common misconceptions related to adding and subtracting fractions with unlike denominators. Understanding these concepts is essential for developing a strong foundation in fractions and their operations.

Question 1: Why is it important to find a common denominator when adding or subtracting fractions with unlike denominators?

Finding a common denominator is crucial because it allows us to combine fractions with different denominators into equivalent fractions with the same denominator. This common denominator serves as the common unit of measurement, enabling us to add or subtract the numerators while keeping the denominator the same.

Question 2: How do I find the least common denominator (LCD) of two or more fractions?

The LCD is the smallest common multiple of the denominators. To find the LCD, list the multiples of each denominator and identify the smallest number that appears in all the lists.

Question 3: What is the rule for adding fractions with unlike denominators?

To add fractions with unlike denominators, convert them to equivalent fractions with a common denominator. Then, add the numerators while keeping the common denominator.

Question 4: What is the rule for subtracting fractions with unlike denominators?

To subtract fractions with unlike denominators, convert them to equivalent fractions with a common denominator. Then, subtract the numerators while keeping the common denominator.

Question 5: How do I simplify fractions after adding or subtracting them?

After adding or subtracting fractions, simplify the resulting fraction by dividing both the numerator and denominator by their greatest common factor (GCF).

Question 6: What are some real-world applications of adding and subtracting fractions with unlike denominators?

Adding and subtracting fractions with unlike denominators is used in various real-world applications, such as cooking, carpentry, engineering, and scientific calculations. For example, in cooking, it is necessary to adjust recipe quantities based on the number of servings, which involves adding or subtracting fractions with unlike denominators.

Summary: Understanding how to add and subtract fractions with unlike denominators is essential for solving problems involving fractional quantities accurately. By mastering these concepts, individuals can develop a strong foundation in fractions and their operations, which is applicable in various fields and everyday life situations.

Transition to the next article section: This understanding of fractions and their operations provides a solid foundation for exploring more advanced mathematical concepts and applications.

Conclusion on Adding and Subtracting Fractions with Unlike Denominators

This exploration of “adding and subtracting fractions with unlike denominators worksheet” has highlighted the significance of understanding how to perform these operations accurately. Fractions are an essential part of mathematics, and the ability to add and subtract them with unlike denominators is crucial for solving problems involving fractional quantities.

Mastering the concepts and techniques discussed in this article provides a solid foundation for further mathematical exploration. By understanding how to find a common denominator, convert fractions to equivalent forms, and simplify fractions, individuals can develop a strong grasp of fractions and their operations. This understanding is applicable in various fields and situations, making it an important skill for students and individuals alike.

Youtube Video: