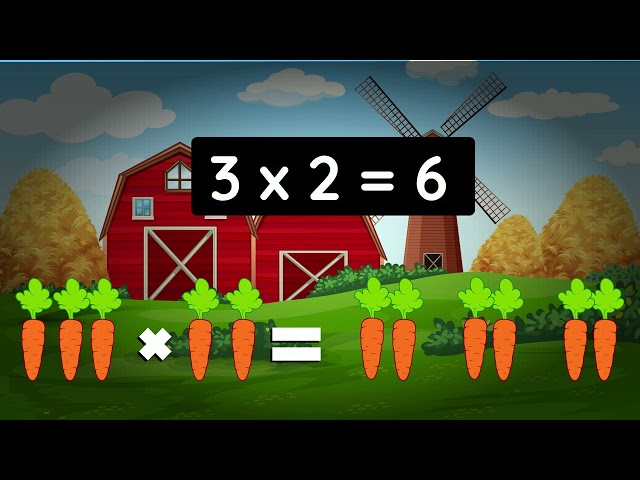Multiplication is Repeated Addition Worksheets Charts and Diagrams
Multiplication is a mathematical operation that involves repeated addition. For example, the multiplication problem 3 4 can be solved by adding 3 four times: 3 + 3 + 3 + 3 = 12. Multiplication is a useful operation that can be used to solve a variety of problems, such as finding the area of a rectangle or the volume of a cube.
There are a variety of charts and diagrams that can be used to help students learn about multiplication. One common type of chart is the multiplication table. A multiplication table is a grid that shows the products of all the numbers from 1 to 12. Multiplication tables can be used to help students memorize the multiplication facts. Another common type of diagram is the array diagram. An array diagram is a visual representation of a multiplication problem. Array diagrams can help students to understand the relationship between multiplication and repeated addition.
Charts and diagrams can be a helpful tool for teaching multiplication. They can help students to visualize the multiplication process and to memorize the multiplication facts. There are a variety of different charts and diagrams that can be used, so it is important to choose the ones that are most appropriate for the students’ needs.
Benefits of using charts and diagrams to teach multiplication:
- Charts and diagrams can help students to visualize the multiplication process.
- Charts and diagrams can help students to memorize the multiplication facts.
- Charts and diagrams can help students to understand the relationship between multiplication and repeated addition.
Conclusion:
Charts and diagrams can be a valuable tool for teaching multiplication. They can help students to learn the multiplication facts, understand the relationship between multiplication and repeated addition, and visualize the multiplication process.
Multiplication is Repeated Addition Worksheets
Multiplication is a mathematical operation that involves repeated addition. For example, the multiplication problem 3 4 can be solved by adding 3 four times: 3 + 3 + 3 + 3 = 12. Multiplication is a useful operation that can be used to solve a variety of problems, such as finding the area of a rectangle or the volume of a cube.
- Visual: Multiplication can be represented visually using arrays or area models.
- Commutative: The order of the factors in a multiplication problem does not matter. For example, 3 4 = 4 3.
- Associative: The grouping of factors in a multiplication problem does not matter. For example, (3 4) 5 = 3 (4 5).
- Distributive: Multiplication can be distributed over addition and subtraction. For example, 3 (4 + 5) = 3 4 + 3 5.
- Inverse: Multiplication and division are inverse operations. This means that you can undo multiplication by dividing. For example, if you multiply 3 by 4 to get 12, you can divide 12 by 4 to get 3.
- Word problems: Multiplication can be used to solve a variety of word problems. For example, if you have 3 baskets with 4 apples in each basket, you can use multiplication to find the total number of apples: 3 4 = 12.
These are just a few of the key aspects of multiplication. By understanding these aspects, students can develop a strong foundation in multiplication and use it to solve a variety of problems.
Visual
Multiplication can be represented visually using arrays or area models. This can help students to understand the concept of multiplication as repeated addition. For example, the multiplication problem 3 x 4 can be represented using an array of 3 rows and 4 columns, or as an area model with a length of 3 and a width of 4.
- Arrays: An array is a rectangular arrangement of objects. In the context of multiplication, an array can be used to represent the factors and the product. For example, the array below represents the multiplication problem 3 x 4:

- Area models: An area model is a rectangle that is divided into smaller rectangles. The length and width of the area model represent the factors of the multiplication problem, and the area of the area model represents the product. For example, the area model below represents the multiplication problem 3 x 4:

Using arrays and area models to represent multiplication can help students to visualize the multiplication process and to understand the relationship between multiplication and repeated addition.
Commutative
The commutative property of multiplication is an important concept for students to understand. It states that the order of the factors in a multiplication problem does not matter. This means that 3 x 4 is the same as 4 x 3. This property can be used to make multiplication problems easier to solve. For example, if a student is having trouble solving the problem 12 x 5, they can use the commutative property to change it to 5 x 12, which may be easier for them to solve.
The commutative property is also important for understanding multiplication as repeated addition. When we multiply two numbers, we are essentially adding one of the numbers to itself the other number of times. For example, 3 x 4 is the same as adding 3 to itself 4 times: 3 + 3 + 3 + 3 = 12. The commutative property tells us that we can add the numbers in any order, so we could also add 4 to itself 3 times to get the same answer: 4 + 4 + 4 = 12.
The commutative property is a valuable tool for solving multiplication problems. It can make problems easier to solve and it can help students to understand the relationship between multiplication and repeated addition.
Here are some real-life examples of the commutative property of multiplication:
- If a farmer has 3 rows of corn with 4 plants in each row, he has the same number of plants as if he had 4 rows of corn with 3 plants in each row.
- If a store sells apples in bags of 3 or bags of 4, the total number of apples sold is the same regardless of which size bag is used.
- If a construction worker needs to lay 3 bricks in 4 different places, he can do this in any order and the result will be the same.
Conclusion:
The commutative property of multiplication is an important concept for students to understand. It can make multiplication problems easier to solve and it can help students to understand the relationship between multiplication and repeated addition.
Associative
The associative property of multiplication is closely related to the concept of multiplication as repeated addition. This property states that the grouping of factors in a multiplication problem does not matter. For example, (3 4) 5 is the same as 3 (4 5). This means that we can group the factors in any way that we want and the result will be the same.
-
Facet 1: Simplifying Complex Multiplication Problems
The associative property can be used to simplify complex multiplication problems. For example, the problem (3 x 4) x 5 can be rewritten as 3 x (4 x 5). This can make the problem easier to solve, especially for students who are still learning multiplication.
-
Facet 2: Understanding the Distributive Property
The associative property is also related to the distributive property. The distributive property states that multiplication can be distributed over addition and subtraction. For example, 3 x (4 + 5) = 3 x 4 + 3 x 5. This property can be used to solve multiplication problems involving parentheses.
-
Facet 3: Applications in Real-Life Situations
The associative property has many applications in real-life situations. For example, a farmer might need to calculate the total number of apples he has by multiplying the number of apples in each basket by the number of baskets. The associative property tells us that the farmer can group the baskets in any way he wants and the total number of apples will be the same.
The associative property of multiplication is an important concept for students to understand. It can help them to simplify multiplication problems, understand the distributive property, and solve multiplication problems involving parentheses. The associative property also has many applications in real-life situations.
Distributive
The distributive property is closely related to the concept of multiplication as repeated addition. This property states that multiplication can be distributed over addition and subtraction. For example, 3 x (4 + 5) = 3 x 4 + 3 x 5. This means that we can multiply a number by a sum or difference and get the same result as if we multiplied the number by each addend or subtrahend separately.
-
Facet 1: Simplifying Multiplication Problems
The distributive property can be used to simplify multiplication problems. For example, the problem 3 x (4 + 5) can be rewritten as 3 x 4 + 3 x 5. This can make the problem easier to solve, especially for students who are still learning multiplication.
-
Facet 2: Solving Multiplication Problems with Parentheses
The distributive property can also be used to solve multiplication problems involving parentheses. For example, the problem (3 + 4) x 5 can be rewritten as 3 x 5 + 4 x 5. This can make the problem easier to solve, especially for students who are still learning multiplication.
-
Facet 3: Applications in Real-Life Situations
The distributive property has many applications in real-life situations. For example, a farmer might need to calculate the total number of apples he has by multiplying the number of apples in each basket by the number of baskets. The distributive property tells us that the farmer can multiply the number of apples in each basket by the total number of baskets, or he can add the number of apples in each basket and then multiply the total by the number of baskets. The result will be the same either way.
The distributive property is an important concept for students to understand. It can help them to simplify multiplication problems, solve multiplication problems involving parentheses, and solve multiplication problems in real-life situations.
Inverse
The inverse relationship between multiplication and division is a fundamental concept in mathematics. It is important for students to understand this relationship in order to be able to solve multiplication and division problems. Multiplication is the process of adding a number to itself a certain number of times. For example, 3 x 4 is the same as adding 3 to itself 4 times: 3 + 3 + 3 + 3 = 12. Division is the inverse operation of multiplication. It is the process of finding out how many times one number is contained within another number. For example, 12 4 is the same as asking how many times 4 goes into 12. The answer is 3, because 4 goes into 12 three times: 4 + 4 + 4 = 12.
The inverse relationship between multiplication and division is a valuable tool for solving multiplication and division problems. For example, if a student is having trouble solving the problem 12 4, they can use the inverse relationship to change it to 4 x ? = 12. This may be easier for them to solve, because they can simply ask themselves what number, when multiplied by 4, equals 12. The answer is 3, so 12 4 = 3.
The inverse relationship between multiplication and division also has many applications in real-life situations. For example, a farmer might need to know how many rows of corn he can plant in his field. He can use multiplication to find the total number of corn plants he needs, and then use division to find out how many rows he can plant with that number of plants.
Understanding the inverse relationship between multiplication and division is an important skill for students to develop. It can help them to solve multiplication and division problems, and to understand how multiplication and division are used in real-life situations.
Word problems
Multiplication is repeated addition. This means that we can use multiplication to solve word problems that involve repeated addition. For example, the word problem above can be solved by adding 4 apples 3 times: 4 + 4 + 4 = 12. However, multiplication is a more efficient way to solve this problem. We can simply multiply 3 by 4 to get the answer: 12.
Word problems are an important part of learning multiplication. They help students to apply their multiplication skills to real-life situations. Multiplication is used in many different areas of life, such as shopping, cooking, and construction. By learning how to solve word problems, students can develop a strong foundation in multiplication that will help them to succeed in school and in life.
Here are some tips for solving word problems involving multiplication:
- Read the problem carefully and identify the key information.
- Determine what operation you need to use to solve the problem.
- Write a multiplication equation to represent the problem.
- Solve the equation to find the answer.
With practice, students can become proficient at solving word problems involving multiplication. This skill will help them to succeed in school and in life.
Multiplication is a mathematical operation that involves repeated addition. For example, the multiplication problem 3 4 can be solved by adding 3 four times: 3 + 3 + 3 + 3 = 12. Multiplication worksheets can be a helpful tool for students to practice their multiplication skills.
Multiplication is an important operation in mathematics. It is used in a variety of applications, such as finding the area of a rectangle, the volume of a cube, and the speed of an object. Multiplication worksheets can help students to develop a strong foundation in multiplication, which will benefit them in their future math studies.
Multiplication is repeated addition worksheets are a valuable resource for students. They can help students to understand the concept of multiplication, practice their multiplication skills, and prepare for future math courses.
Frequently Asked Questions about Multiplication as Repeated Addition Worksheets
Multiplication is repeated addition worksheets are a valuable resource for students to practice their multiplication skills and develop a strong foundation in mathematics. Here are some frequently asked questions about multiplication as repeated addition worksheets:
Question 1: What is the purpose of multiplication as repeated addition worksheets?
Multiplication as repeated addition worksheets help students to understand the concept of multiplication as repeated addition. They provide practice in solving multiplication problems using this method, which can help students to develop a strong foundation in multiplication.
Question 2: What are the benefits of using multiplication as repeated addition worksheets?
Multiplication as repeated addition worksheets can help students to:
- Understand the concept of multiplication as repeated addition
- Develop fluency in solving multiplication problems
- Build a strong foundation in multiplication
Question 3: How can I use multiplication as repeated addition worksheets in my classroom?
Multiplication as repeated addition worksheets can be used in a variety of ways in the classroom. They can be used as:
- Independent practice
- Guided practice
- Assessment
Question 4: What are some tips for using multiplication as repeated addition worksheets?
Here are some tips for using multiplication as repeated addition worksheets:
- Start with simple problems and gradually increase the difficulty.
- Provide students with manipulatives, such as counters or blocks, to help them visualize the multiplication process.
- Encourage students to explain their thinking as they solve the problems.
Question 5: Where can I find multiplication as repeated addition worksheets?
There are many places where you can find multiplication as repeated addition worksheets. You can find them online, in teacher supply stores, and in some textbooks.
Question 6: Are there any other resources available to help students learn about multiplication as repeated addition?
In addition to multiplication as repeated addition worksheets, there are a variety of other resources available to help students learn about multiplication as repeated addition. These resources include:
- Books
- Websites
- Games
- Videos
Summary of key takeaways or final thought:
Multiplication as repeated addition worksheets are a valuable resource for students to practice their multiplication skills and develop a strong foundation in mathematics. By using these worksheets, students can learn to understand the concept of multiplication as repeated addition, develop fluency in solving multiplication problems, and build a strong foundation in multiplication.
Transition to the next article section:
For more information on multiplication as repeated addition, please see the following resources:
- Multiplication as Repeated Addition Worksheets
- Benefits of Multiplication as Repeated Addition Worksheets
- Tips for Using Multiplication as Repeated Addition Worksheets
Conclusion
Multiplication is repeated addition worksheets are a valuable resource for students to practice their multiplication skills and develop a strong foundation in mathematics. These worksheets help students to understand the concept of multiplication as repeated addition, develop fluency in solving multiplication problems, and build a strong foundation in multiplication. By using these worksheets, students can become proficient in multiplication, which will benefit them in their future math studies and in their everyday lives.
Multiplication is an essential mathematical operation that is used in a variety of applications. By understanding the concept of multiplication as repeated addition, students can develop a strong foundation in multiplication that will help them to succeed in school and in life.
Youtube Video: