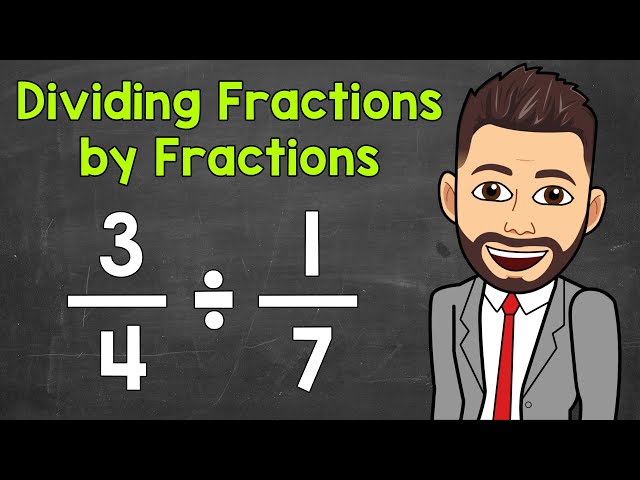
Dividing Fractions by Fractions Worksheet
This worksheet provides practice in dividing fractions by fractions. It is a valuable tool for students who are learning this important skill. The worksheet includes a variety of problems, ranging from simple to complex. It also includes step-by-step instructions on how to solve each problem. This worksheet is a great way for students to master the skill of dividing fractions by fractions.
Examples of dividing fractions by fractions worksheets
- Dividing Fractions by Fractions Practice Worksheet from Math-Aids.com
- Dividing Fractions by Fractions Worksheet from Khan Academy
- Dividing Fractions by Fractions Worksheet from Kuta Software
Steps to dividing fractions by fractions worksheet creation
- Start with the basics. Make sure that students understand how to multiply and divide fractions before moving on to dividing fractions by fractions.
- Introduce the concept of dividing fractions by fractions. Explain that when you divide a fraction by a fraction, you are essentially multiplying the first fraction by the reciprocal of the second fraction.
- Provide students with practice problems. Start with simple problems and gradually increase the difficulty as students progress.
- Check students’ work. Make sure that students are understanding the concepts and are able to apply them correctly.
Benefits of dividing fractions by fractions worksheet
- Improves students’ understanding of fractions.
- Develops students’ problem-solving skills.
- Prepares students for more advanced math topics.
Dividing fractions by fractions is an important skill for students to master. This worksheet provides a valuable tool for students to practice this skill and improve their understanding of fractions.
Dividing Fractions by Fractions Worksheet
Dividing fractions by fractions is an important skill in mathematics. It is used in a variety of applications, such as solving proportions, scaling recipes, and calculating rates. To master this skill, it is essential to understand the key aspects of dividing fractions by fractions.
- Definition: Dividing a fraction by a fraction is the same as multiplying the first fraction by the reciprocal of the second fraction.
- Reciprocal: The reciprocal of a fraction is the fraction flipped upside down. For example, the reciprocal of 1/2 is 2/1.
- Invert and Multiply: To divide fractions, invert the second fraction and multiply. For example, to divide 1/2 by 1/4, you would invert 1/4 to get 4/1 and then multiply 1/2 by 4/1, which gives you 4/2 or 2.
- Simplify: After multiplying the fractions, simplify the answer by reducing it to its lowest terms. For example, if you get 4/8 as your answer, you would simplify it to 1/2.
- Applications: Dividing fractions by fractions is used in a variety of applications, such as solving proportions, scaling recipes, and calculating rates.
- Practice: The best way to master dividing fractions by fractions is to practice. There are many online resources and worksheets available to help you practice this skill.
These are just a few of the essential aspects of dividing fractions by fractions. By understanding these concepts, you will be able to master this skill and use it to solve a variety of problems.
Definition
This definition is the foundation for understanding how to divide fractions by fractions. It states that when you divide a fraction by a fraction, you are essentially multiplying the first fraction by the reciprocal of the second fraction. This is because the reciprocal of a fraction is the fraction flipped upside down. For example, the reciprocal of 1/2 is 2/1. When you multiply a fraction by its reciprocal, you get 1. This is because multiplying any number by its reciprocal is the same as dividing that number by itself, which always equals 1.
Dividing fractions by fractions worksheets are essential for practicing this skill. These worksheets provide students with a variety of problems to solve, ranging from simple to complex. By completing these worksheets, students can develop a strong understanding of how to divide fractions by fractions and how they can use this skill to solve real-world problems.
For example, dividing fractions by fractions is used in a variety of applications, such as:
- Solving proportions
- Scaling recipes
- Calculating rates
By understanding the definition of dividing fractions by fractions and practicing this skill on worksheets, students will be prepared to use this skill in a variety of real-world applications.
In conclusion, the definition of dividing fractions by fractions is the foundation for understanding how to solve this type of problem. Dividing fractions by fractions worksheets are essential for practicing this skill and developing a strong understanding of how to use it to solve real-world problems.
Reciprocal
The concept of a reciprocal is essential for understanding how to divide fractions by fractions. A reciprocal is simply a fraction that has been flipped upside down. For example, the reciprocal of 1/2 is 2/1. This means that 1/2 multiplied by 2/1 is equal to 1. This is because multiplying any number by its reciprocal is the same as dividing that number by itself, which always equals 1.
When dividing fractions by fractions, we use the reciprocal of the second fraction. For example, to divide 1/2 by 1/4, we would invert 1/4 to get 4/1 and then multiply 1/2 by 4/1. This gives us the answer of 2.
Dividing fractions by fractions worksheets are essential for practicing this skill. These worksheets provide students with a variety of problems to solve, ranging from simple to complex. By completing these worksheets, students can develop a strong understanding of how to divide fractions by fractions and how to use this skill to solve real-world problems.
For example, dividing fractions by fractions is used in a variety of applications, such as:
- Solving proportions
- Scaling recipes
- Calculating rates
By understanding the concept of a reciprocal and practicing this skill on worksheets, students will be prepared to use this skill in a variety of real-world applications.
In conclusion, the concept of a reciprocal is essential for understanding how to divide fractions by fractions. Dividing fractions by fractions worksheets are essential for practicing this skill and developing a strong understanding of how to use it to solve real-world problems.
Invert and Multiply
The “invert and multiply” rule is a fundamental concept in dividing fractions. It is essential for understanding how to solve this type of problem. By inverting the second fraction and multiplying, we are essentially converting the division problem into a multiplication problem. This makes it much easier to solve.
Dividing fractions by fractions worksheets are essential for practicing this skill. These worksheets provide students with a variety of problems to solve, ranging from simple to complex. By completing these worksheets, students can develop a strong understanding of how to use the “invert and multiply” rule to divide fractions by fractions.
For example, dividing fractions by fractions is used in a variety of applications, such as:
- Solving proportions
- Scaling recipes
- Calculating rates
By understanding the “invert and multiply” rule and practicing this skill on worksheets, students will be prepared to use this skill in a variety of real-world applications.
In conclusion, the “invert and multiply” rule is a fundamental concept in dividing fractions by fractions. Dividing fractions by fractions worksheets are essential for practicing this skill and developing a strong understanding of how to use it to solve real-world problems.
Simplify
Simplifying fractions is an essential part of dividing fractions by fractions. After multiplying the fractions, we need to simplify the answer by reducing it to its lowest terms. This means finding the greatest common factor (GCF) of the numerator and denominator and dividing both by the GCF. For example, if we get 4/8 as our answer, we can simplify it to 1/2 by dividing both the numerator and denominator by 2. This gives us the simplest form of the fraction, which is the fraction with the smallest possible numerator and denominator.
Dividing fractions by fractions worksheets are essential for practicing this skill. These worksheets provide students with a variety of problems to solve, ranging from simple to complex. By completing these worksheets, students can develop a strong understanding of how to simplify fractions and how to use this skill to solve real-world problems.
For example, simplifying fractions is used in a variety of applications, such as:
- Solving proportions
- Scaling recipes
- Calculating rates
By understanding how to simplify fractions and practicing this skill on worksheets, students will be prepared to use this skill in a variety of real-world applications.
In conclusion, simplifying fractions is an essential part of dividing fractions by fractions. Dividing fractions by fractions worksheets are essential for practicing this skill and developing a strong understanding of how to use it to solve real-world problems.
Applications
Dividing fractions by fractions is a fundamental skill in mathematics with a wide range of applications. Understanding these applications is crucial for students to grasp the significance of mastering this skill.
One important application of dividing fractions by fractions is solving proportions. Proportions are equations that state that two ratios are equal. For example, the proportion 1/2 = 2/4 states that the ratio of 1 to 2 is equal to the ratio of 2 to 4. Solving proportions is essential in many areas, such as chemistry, physics, and engineering.
Another application of dividing fractions by fractions is scaling recipes. When scaling a recipe up or down, we need to adjust the amount of each ingredient . For example, if we want to double a recipe, we need to multiply the amount of each ingredient by 2. However, if we want to halve a recipe, we need to divide the amount of each ingredient by 2. Dividing fractions by fractions is essential for scaling recipes accurately.
Finally, dividing fractions by fractions is also used in calculating rates. A rate is a measure of the change in one quantity relative to the change in another quantity. For example, speed is a rate that measures the change in distance relative to the change in time. Calculating rates is essential in many areas, such as economics, finance, and sports.
In conclusion, dividing fractions by fractions is a fundamental skill with a wide range of applications. Understanding these applications is crucial for students to grasp the significance of mastering this skill and to appreciate its practical value in real-world scenarios.
Practice
Practice is essential for mastering any skill, and dividing fractions by fractions is no exception. The best way to improve your skills in this area is to practice regularly. There are many online resources and worksheets available that can help you practice this skill.
Dividing fractions by fractions worksheets are a valuable tool for practicing this skill. These worksheets provide students with a variety of problems to solve, ranging from simple to complex. By completing these worksheets, students can develop a strong understanding of how to divide fractions by fractions and how to use this skill to solve real-world problems.
For example, dividing fractions by fractions is used in a variety of applications, such as:
- Solving proportions
- Scaling recipes
- Calculating rates
By understanding how to divide fractions by fractions and practicing this skill on worksheets, students will be prepared to use this skill in a variety of real-world applications.
In conclusion, practice is essential for mastering dividing fractions by fractions. Dividing fractions by fractions worksheets are a valuable tool for practicing this skill. By completing these worksheets, students can develop a strong understanding of how to divide fractions by fractions and how to use this skill to solve real-world problems.
A worksheet on dividing fractions by fractions is an educational tool that provides practice in performing the mathematical operation of dividing one fraction by another. Fractions represent parts of a whole, and dividing fractions involves finding a fraction that, when multiplied by the divisor fraction, results in the dividend fraction.
Dividing fractions by fractions is a fundamental skill in mathematics, with applications in various fields such as science, engineering, and finance. It is essential for solving problems involving ratios, proportions, and rates. Historically, dividing fractions has been a crucial concept in mathematics, with mathematicians developing algorithms and techniques to simplify and solve these calculations.
This article will delve into the significance and benefits of “dividing fractions by fractions worksheets,” exploring their role in developing mathematical proficiency and problem-solving skills. We will also discuss strategies for effectively using these worksheets to enhance understanding and foster a deeper grasp of this mathematical operation.
FAQs on Dividing Fractions by Fractions Worksheets
Dividing fractions by fractions is a fundamental mathematical operation that requires practice and understanding. Worksheets are valuable tools for developing proficiency in this skill. Here we address some frequently asked questions about dividing fractions by fractions worksheets:
Question 1: What is the purpose of dividing fractions by fractions worksheets?
Dividing fractions by fractions worksheets provide guided practice for students to improve their skills in performing this mathematical operation. They offer a structured approach to learning and reinforcing concepts.
Question 2: Who can benefit from using dividing fractions by fractions worksheets?
These worksheets are suitable for students at various levels, from those learning the concept for the first time to those who need extra practice. They can be used in classrooms, for homework assignments, or as self-study resources.
Question 3: What types of problems are typically found on dividing fractions by fractions worksheets?
Worksheets may include a range of problems, from basic division of simple fractions to more complex problems involving mixed numbers and improper fractions. They may also incorporate real-world scenarios to enhance practical understanding.
Question 4: How can I effectively use dividing fractions by fractions worksheets?
To maximize learning, it’s recommended to approach worksheets systematically. Start with simpler problems, gradually increasing the difficulty as you progress. Ensure a clear understanding of each step, and seek assistance if needed.
Question 5: Are there any online resources for dividing fractions by fractions worksheets?
Yes, there are numerous online resources that provide access to printable dividing fractions by fractions worksheets. These worksheets often come with answer keys for self-assessment and can be a convenient tool for practice.
Question 6: How do dividing fractions by fractions worksheets contribute to mathematical proficiency?
Regular practice with dividing fractions by fractions worksheets strengthens students’ understanding of fractions, develops their problem-solving abilities, and prepares them for more advanced mathematical concepts.
In summary, dividing fractions by fractions worksheets are valuable resources for enhancing mathematical proficiency. By providing structured practice, they help students develop a solid foundation in this essential operation.
Transition to the next article section: Understanding the significance and benefits of dividing fractions by fractions worksheets is crucial. In the next section, we will explore strategies for using these worksheets effectively to maximize learning outcomes.
Conclusion
In conclusion, “dividing fractions by fractions worksheets” play a pivotal role in developing mathematical proficiency. They provide a structured and effective approach to practicing and mastering this fundamental operation.
Through consistent engagement with these worksheets, students can solidify their understanding of fractions, enhance their problem-solving skills, and prepare themselves for more complex mathematical concepts. The significance of these worksheets lies in their ability to foster a deeper comprehension of the mathematical principles involved in dividing fractions by fractions.
Youtube Video: