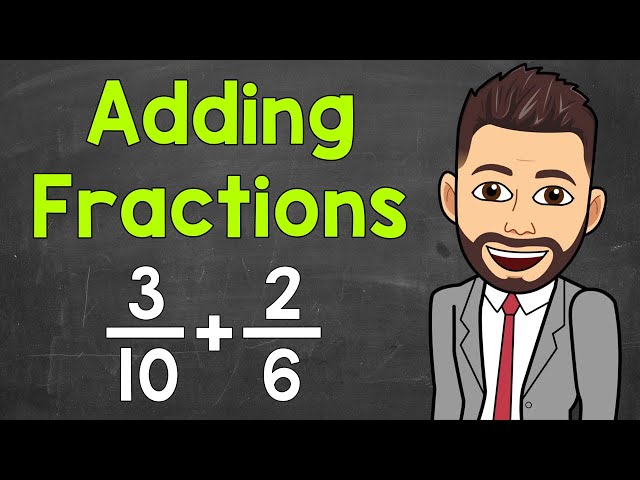
Adding Fractions with Different Denominators Worksheet
This worksheet has two sections. The first section includes 10 multiple-choice questions in which students are asked to add two fractions with different denominators. The second section includes 10 free-response questions in which students are asked to add three or more fractions with different denominators.
Steps to Add Fractions with Different Denominators:
- Find the Least Common Multiple (LCM) of the denominators. The LCM is the smallest number that is divisible by all of the denominators.
- Multiply the numerator and denominator of each fraction by the LCM of the denominators. This will create equivalent fractions with the same denominator.
- Add the numerators of the equivalent fractions. The denominator remains the same.
- Simplify the fraction, if possible.
Benefits of Using a Worksheet to Practice Adding Fractions with Different Denominators:
- Provides students with an opportunity to practice the skill of adding fractions with different denominators.
- Helps students to develop their understanding of equivalent fractions.
- Can be used as an assessment tool to track student progress.
Conclusion:Adding fractions with different denominators can be a challenging skill for students to master. However, with practice, students can become proficient in this skill. This worksheet provides students with an opportunity to practice adding fractions with different denominators in a variety of ways.
Essential Aspects of Adding Fractions with Different Denominators Worksheet
Adding fractions with different denominators can be a challenging concept for students to grasp. However, by understanding the essential aspects of this topic, students can develop a strong foundation in this skill. Some key aspects to consider include:
- Definition: Adding fractions with different denominators involves finding a common denominator, multiplying the numerator and denominator of each fraction by the appropriate factor, and then adding the numerators.
- Steps: The steps involved in adding fractions with different denominators include finding the least common multiple of the denominators, multiplying the numerator and denominator of each fraction by the appropriate factor, and then adding the numerators.
- Common Denominator: A common denominator is a multiple of all the denominators in the fractions being added.
- Equivalent Fractions: Equivalent fractions are fractions that have the same value, even though they may have different numerators and denominators.
- Simplifying Fractions: Simplifying fractions involves dividing the numerator and denominator by their greatest common factor.
- Applications: Adding fractions with different denominators is a skill that is used in a variety of applications, such as cooking, construction, and science.
- Challenges: Students may encounter challenges when adding fractions with different denominators, such as finding the least common multiple and simplifying fractions.
- Instruction: Effective instruction in adding fractions with different denominators includes providing clear explanations, using visual aids, and providing opportunities for practice.
By understanding these key aspects, students can develop a strong foundation in adding fractions with different denominators. This skill is essential for success in mathematics and in a variety of real-world applications.
Definition
This definition is the foundation for understanding how to add fractions with different denominators. It provides a step-by-step process that can be applied to any two fractions, regardless of their denominators.
- Finding a common denominator is the first step in adding fractions with different denominators. The common denominator is the least common multiple of the two denominators. This means that it is the smallest number that is divisible by both denominators.
- Multiplying the numerator and denominator of each fraction by the appropriate factor is the second step in adding fractions with different denominators. The appropriate factor is the number that makes the denominator of each fraction equal to the common denominator.
- Adding the numerators is the third and final step in adding fractions with different denominators. Once the denominators of the two fractions are equal, the numerators can be added together. The sum of the numerators is the numerator of the new fraction.
These steps are essential for understanding how to add fractions with different denominators. By following these steps, students can develop a strong foundation in this skill.
Steps
The steps involved in adding fractions with different denominators are essential for completing “adding fractions with different denominators worksheets”. These worksheets provide students with practice adding fractions with different denominators, and the steps provide a structured approach for solving these problems.
- Finding the least common multiple (LCM) of the denominators is the first step in adding fractions with different denominators. The LCM is the smallest number that is divisible by all of the denominators. This can be found by listing the multiples of each denominator and finding the smallest number that is common to all of the lists.
- Multiplying the numerator and denominator of each fraction by the appropriate factor is the second step in adding fractions with different denominators. The appropriate factor is the number that makes the denominator of each fraction equal to the LCM. This can be found by dividing the LCM by the denominator of each fraction.
- Adding the numerators is the third and final step in adding fractions with different denominators. Once the denominators of the two fractions are equal, the numerators can be added together. The sum of the numerators is the numerator of the new fraction.
By following these steps, students can develop a strong foundation in adding fractions with different denominators. These skills are essential for success in mathematics and in a variety of real-world applications.
Common Denominator
Finding a common denominator is a crucial step in adding fractions with different denominators. It allows us to add the numerators of the fractions, which is the essential operation in fraction addition. In the context of “adding fractions with different denominators worksheets,” understanding the concept of a common denominator is fundamental for completing these worksheets accurately.
-
Role of Common Denominator:
A common denominator serves as the common base upon which different fractions can be added. By finding the least common multiple of the denominators, we create a common unit that allows us to compare and combine the fractions. -
Real-Life Examples:
In real-life situations, finding a common denominator is essential in various applications. For instance, when comparing the speeds of two vehicles traveling at different units (e.g., miles per hour and kilometers per hour), we need to find a common denominator (e.g., kilometers per hour) to compare their speeds accurately. -
Implications for Worksheets:
In “adding fractions with different denominators worksheets,” students are often required to find the common denominator before adding the fractions. This step is crucial for ensuring that the addition is performed correctly and that the resulting fraction is in its simplest form. -
Benefits of Understanding Common Denominator:
Understanding the concept of a common denominator not only helps students complete worksheets but also develops their overall understanding of fractions and their operations. It provides a foundation for more complex operations involving fractions, such as subtraction, multiplication, and division.
In conclusion, the concept of a common denominator is central to adding fractions with different denominators. By understanding the role, examples, and implications of finding a common denominator, students can effectively complete “adding fractions with different denominators worksheets” and develop a strong foundation in fraction operations.
Equivalent Fractions
In the context of “adding fractions with different denominators worksheets,” understanding equivalent fractions is crucial for solving these worksheets accurately and efficiently. When adding fractions with different denominators, we often need to convert them into equivalent fractions with a common denominator before performing the addition. This is where the concept of equivalent fractions comes into play.
-
Role of Equivalent Fractions:
Equivalent fractions allow us to represent the same quantity using different fractions. By converting fractions into equivalent fractions with a common denominator, we can add them more easily and obtain the correct result. -
Real-Life Examples:
In real-life situations, equivalent fractions are used in various applications. For instance, when comparing the prices of different products sold in different units (e.g., ounces and pounds), we can convert them into equivalent fractions with a common unit (e.g., ounces) to compare their prices accurately. -
Implications for Worksheets:
In “adding fractions with different denominators worksheets,” students are often required to convert fractions into equivalent fractions before adding them. This step is essential for ensuring that the addition is performed correctly and that the resulting fraction is in its simplest form. -
Benefits of Understanding Equivalent Fractions:
Understanding the concept of equivalent fractions not only helps students complete worksheets but also develops their overall understanding of fractions and their operations. It provides a foundation for more complex operations involving fractions, such as subtraction, multiplication, and division.
In conclusion, the concept of equivalent fractions is closely linked to “adding fractions with different denominators worksheets.” By understanding the role, examples, and implications of equivalent fractions, students can effectively complete these worksheets and develop a strong foundation in fraction operations.
Simplifying Fractions
Simplifying fractions is an essential skill in mathematics, particularly when working with fractions with different denominators. In the context of “adding fractions with different denominators worksheets”, simplifying fractions plays a crucial role in ensuring that the addition is performed correctly and efficiently.
-
Role in Adding Fractions:
Simplifying fractions before adding them helps to eliminate any common factors between the numerator and denominator, resulting in fractions that are in their simplest form. This makes it easier to find a common denominator and add the fractions accurately. -
Real-Life Examples:
Simplifying fractions has practical applications in various real-life situations. For instance, when comparing the prices of different products sold in different units (e.g., ounces and pounds), simplifying the fractions representing their prices allows for easier comparison and determination of the better value. -
Implications for Worksheets:
In “adding fractions with different denominators worksheets”, students are often required to simplify fractions before adding them. This step is crucial for ensuring that the addition is performed correctly and that the resulting fraction is in its simplest form. -
Benefits of Simplifying Fractions:
Simplifying fractions not only helps students complete worksheets but also develops their overall understanding of fractions and their operations. It provides a foundation for more complex operations involving fractions, such as subtraction, multiplication, and division.
In conclusion, simplifying fractions is an integral part of “adding fractions with different denominators worksheets.” By understanding the role, examples, and implications of simplifying fractions, students can effectively complete these worksheets and develop a strong foundation in fraction operations.
Applications
Adding fractions with different denominators is a fundamental skill used in various practical applications across different fields. Understanding how this skill is applied in real-life scenarios can enhance the learning experience and highlight its importance in “adding fractions with different denominators worksheets”.
In cooking, for instance, recipes often involve precise measurements of ingredients. When scaling a recipe up or down, it becomes necessary to add fractions with different denominators to adjust the quantities accurately. This ensures that the dish retains its intended flavor and consistency.
Similarly, in construction, architects and engineers must calculate measurements and dimensions involving fractions with different denominators. Adding these fractions correctly is crucial for ensuring structural integrity and safety in buildings and infrastructure.
In science, particularly in chemistry, adding fractions with different denominators is essential for determining concentrations of solutions, calculating reaction yields, and analyzing experimental data. Accurate calculations require the ability to add fractions with different denominators efficiently.
By incorporating real-life applications into “adding fractions with different denominators worksheets”, students can grasp the practical significance of this skill and its relevance beyond the classroom. It helps them develop a deeper understanding of the subject and motivates them to master the concepts.
Furthermore, recognizing the applications of adding fractions with different denominators empowers students to appreciate the interconnectedness of mathematics with other disciplines. It fosters a holistic learning approach and encourages students to explore the practical implications of their mathematical knowledge.
In conclusion, understanding the applications of adding fractions with different denominators is an integral part of “adding fractions with different denominators worksheets”. By integrating real-life examples and highlighting the practical significance of this skill, educators can make learning more engaging and meaningful for students.
Challenges
In the context of “adding fractions with different denominators worksheets”, it is crucial to recognize the challenges that students may face when working with fractions with different denominators. Understanding these challenges and incorporating strategies to address them enhances the effectiveness of these worksheets.
-
Finding the Least Common Multiple:
Finding the least common multiple (LCM) of the denominators is a fundamental step in adding fractions with different denominators. However, students may struggle to identify the LCM, especially when dealing with larger numbers. This can lead to errors in finding the common denominator and, consequently, incorrect answers. -
Simplifying Fractions:
Simplifying fractions involves dividing both the numerator and denominator by their greatest common factor. While the concept is straightforward, students may overlook this step or make errors in simplifying the fractions correctly. This can result in fractions that are not in their simplest form, affecting the accuracy of the addition.
To address these challenges, “adding fractions with different denominators worksheets” should incorporate strategies such as:
- Providing clear instructions and examples on how to find the LCM using different methods, such as prime factorization or listing multiples.
- Including practice problems that focus specifically on finding the LCM to strengthen students’ understanding and fluency.
- Emphasizing the importance of simplifying fractions before adding them and providing guidelines on how to identify and divide by the greatest common factor.
By incorporating these strategies, “adding fractions with different denominators worksheets” can help students overcome the challenges associated with adding fractions with different denominators. It allows them to develop a strong foundation in this essential mathematical skill, which is crucial for success in further mathematical concepts and real-life applications.
Instruction
Effective instruction is essential for students to develop a strong understanding of adding fractions with different denominators. By providing clear explanations, using visual aids, and providing opportunities for practice, educators can create a supportive learning environment that fosters student success.
-
Providing Clear Explanations:
Clear explanations are crucial for students to grasp the concepts and procedures involved in adding fractions with different denominators. Teachers should break down the steps involved, using precise language and providing examples to illustrate each step. They should also address common misconceptions and provide strategies for overcoming challenges. -
Using Visual Aids:
Visual aids, such as diagrams, charts, and manipulatives, can greatly enhance students’ understanding of adding fractions with different denominators. These aids can help students visualize the concepts, identify patterns, and make connections between different representations of fractions. By incorporating visual aids into their instruction, teachers can make learning more accessible and engaging. -
Providing Opportunities for Practice:
Practice is essential for students to develop fluency and confidence in adding fractions with different denominators. Educators should provide ample opportunities for students to practice these skills through problem-solving activities, worksheets, and games. By engaging in repeated practice, students can reinforce their understanding and develop the automaticity necessary for success.
Incorporating these instructional strategies into “adding fractions with different denominators worksheets” can significantly enhance their effectiveness. By providing clear explanations, using visual aids, and providing opportunities for practice, these worksheets can help students develop a deep understanding of this important mathematical concept.
In the realm of mathematics, fractions play a pivotal role in representing parts of a whole. When adding fractions with different denominators, a crucial skill for mathematical proficiency, students often encounter challenges. To address this, “adding fractions with different denominators worksheets” emerge as invaluable tools for educators.
These worksheets provide a structured andapproach to guide students through the intricacies of adding fractions with different denominators. By engaging in these exercises, students develop a deep understanding of the concepts involved, fostering their mathematical growth. The worksheets present a variety of problems that require students to apply their knowledge and skills to solve them, ultimately enhancing their problem-solving abilities.
The benefits of utilizing “adding fractions with different denominators worksheets” are multifaceted. Not only do they strengthen students’ understanding of fractions, but they also lay the foundation for more complex mathematical operations involving fractions, such as subtraction, multiplication, and division. Moreover, these worksheets contribute to the development of critical thinking and logical reasoning skills, which are essential for success in various academic disciplines and real-world applications.
FAQs on Adding Fractions with Different Denominators
This FAQ section addresses common questions and misconceptions related to adding fractions with different denominators, providing clear and informative answers to enhance understanding.
Question 1: Why is it necessary to find a common denominator when adding fractions with different denominators?
Answer: Finding a common denominator is essential because it provides a common base for the fractions to be added. It allows the numerators to be added while maintaining the value of the fraction.
Question 2: How do I find the least common multiple (LCM) of the denominators?
Answer: The LCM is the smallest common multiple of the denominators. It can be found by listing the multiples of each denominator and identifying the smallest number that appears in all the lists.
Question 3: What if the denominators do not have any common factors?
Answer: In such cases, the LCM is the product of the denominators. This ensures that both fractions have an equivalent fraction with the same denominator.
Question 4: How do I simplify fractions before adding them?
Answer: Simplifying fractions involves dividing both the numerator and denominator by their greatest common factor. This results in a fraction that is in its simplest form.
Question 5: Can I add fractions with different denominators in my head?
Answer: Adding fractions with different denominators mentally can be challenging but is possible with practice. One method is to find a common denominator in your head and then add the numerators.
Question 6: When are skills in adding fractions with different denominators useful in real life?
Answer: Adding fractions with different denominators is a fundamental skill used in various practical applications, such as cooking, construction, and science, where precise measurements and calculations are necessary.
Summary: Understanding how to add fractions with different denominators is crucial for mathematical proficiency. By addressing common questions and misconceptions, this FAQ section clarifies the concepts involved and provides strategies for solving these problems accurately. Regular practice and a thorough understanding of the principles discussed in this FAQ will empower learners to confidently navigate fractions with different denominators.
Transition to the Next Section: To enhance your understanding further, explore the following resources that provide additional insights into adding fractions with different denominators.
Conclusion
In conclusion, “adding fractions with different denominators worksheets” are invaluable tools for developing a comprehensive understanding of fractions and their operations. Through these worksheets, students engage with various exercises that challenge them to add fractions with different denominators, fostering their problem-solving abilities and critical thinking skills.
By mastering the techniques of finding common denominators, simplifying fractions, and applying appropriate strategies, learners gain confidence in manipulating fractions with different denominators. This proficiency extends beyond the classroom, equipping them to tackle real-world applications that require precise calculations involving fractions, such as in cooking, construction, and scientific research.
As students progress in their mathematical journey, a solid foundation in adding fractions with different denominators serves as a stepping stone to more complex operations and concepts. It empowers them to approach fractions with confidence and enthusiasm, unraveling the intricacies of this fundamental mathematical concept.
Youtube Video: