Master Division with Free 3rd Grade Division Worksheets

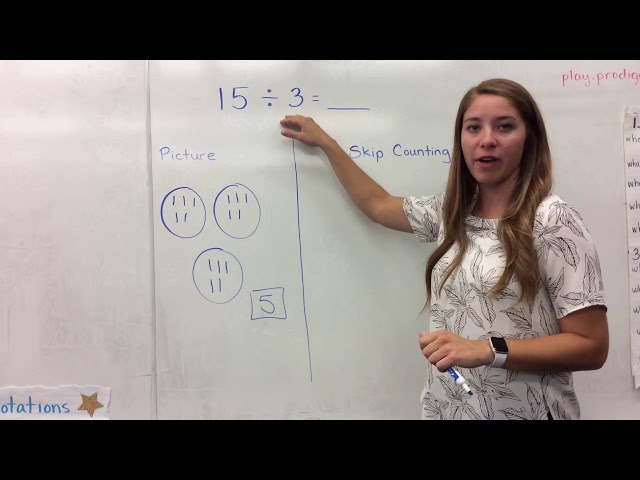
Diagrams and Charts for “3rd Grade Division Problems”

Visual aids can be powerful tools for teaching and learning division. They can help students to understand the concepts involved, and to see how division works in practice. By incorporating diagrams and charts into third-grade math instruction, teachers can make learning division more engaging and effective.

There are many different types of diagrams and charts that can be used to teach third-grade division problems. Some common examples include:

Division number line: A number line can be used to illustrate the process of division. To divide a number by another number, students can start at the dividend and count back by the divisor until they reach the quotient.
Array model: An array model can be used to represent a division problem as a rectangle. The length of the rectangle represents the dividend, the width of the rectangle represents the divisor, and the area of the rectangle represents the quotient.
Repeated subtraction: Repeated subtraction can be used to solve division problems. To divide a number by another number, students can repeatedly subtract the divisor from the dividend until there is no remainder.

When creating diagrams and charts to teach division, it is important to keep the following guidelines in mind:

Use clear and concise visuals. Diagrams and charts should be easy for students to understand. Avoid using too much clutter or unnecessary details.
Label all parts of the diagram or chart. This will help students to identify the different parts of the division problem.
Use color to highlight important information. Color can be used to draw attention to the quotient, remainder, or other important parts of the division problem.

Diagrams and charts can be a valuable tool for teaching third-grade division problems. By incorporating these visual aids into instruction, teachers can make learning division more engaging and effective.

Essential Aspects of 3rd Grade Division Problems

Division is a fundamental operation in mathematics that involves splitting a quantity into equal parts. In third grade, students begin to learn the concept of division and how to solve division problems. There are several key aspects of third grade division problems that are essential for students to understand.

Dividend: The number being divided.
Divisor: The number by which the dividend is being divided.
Quotient: The answer to a division problem.
Remainder: The amount left over after dividing the dividend by the divisor.
Division algorithm: The step-by-step process used to solve division problems.
Division strategies: Mental math strategies that can be used to solve division problems.
Word problems: Real-world problems that can be solved using division.

These key aspects are all interconnected and essential for understanding division. For example, students need to understand the concept of the dividend and divisor in order to solve division problems using the division algorithm. They also need to be able to recognize and interpret remainders. Division strategies can help students to solve division problems more efficiently, and word problems provide a context for students to apply their division skills to real-world situations.

By understanding these key aspects of third grade division problems, students can develop a strong foundation in division that will help them to succeed in mathematics.

Dividend

The dividend is a crucial component of third grade division problems. It represents the total quantity that is being divided into equal parts. Understanding the concept of the dividend is essential for students to be able to solve division problems accurately and efficiently.

In real-world situations, the dividend can represent a variety of quantities. For example, if a farmer has 24 apples and wants to divide them equally among 4 baskets, the dividend would be 24. The divisor would be 4, and the quotient would be 6. This means that each basket would have 6 apples.

The dividend can also be a fraction. For example, if a teacher wants to divide 1/2 of a pizza equally among 3 students, the dividend would be 1/2. The divisor would be 3, and the quotient would be 1/6. This means that each student would get 1/6 of the pizza.

Understanding the concept of the dividend is essential for students to be able to solve division problems in a variety of contexts. By recognizing the dividend as the total quantity that is being divided, students can develop a deeper understanding of the division process.

Divisor

In third grade division problems, the divisor plays a critical role in determining the number of equal parts that the dividend will be divided into. Understanding the concept of the divisor is essential for students to be able to solve division problems accurately and efficiently.

Determining the number of groups: The divisor represents the number of equal groups that the dividend will be divided into. For example, if a farmer has 24 apples and wants to divide them equally among 4 baskets, the divisor would be 4. This means that the apples will be divided into 4 equal groups.
Finding the size of each group: The divisor also helps to determine the size of each group. In the previous example, since the divisor is 4, each basket will have 24 / 4 = 6 apples.
Relating to multiplication: The divisor is closely related to multiplication. The dividend can be thought of as the product of the divisor and the quotient. For example, in the previous example, the dividend is 24, the divisor is 4, and the quotient is 6. This can be expressed as the multiplication equation 4 x 6 = 24.
Using estimation: The divisor can be used to estimate the quotient. For example, if a student is dividing 24 by 4, they can estimate that the quotient will be around 6. This is because 4 goes into 24 about 6 times.

Understanding the concept of the divisor is essential for students to be able to solve division problems in a variety of contexts. By recognizing the divisor as the number that determines the number and size of equal groups, students can develop a deeper understanding of the division process.

Quotient

In the realm of third-grade division problems, the quotient occupies a central position as the ultimate outcome of the division process. Understanding the concept of the quotient is crucial for students to grasp the essence of division and solve division problems with accuracy and efficiency.

Numerical Representation: The quotient is the numerical value that expresses the number of equal groups or the number of times the divisor is contained within the dividend. For instance, if a farmer divides 24 apples equally among 4 baskets, the quotient would be 6, indicating that each basket receives 6 apples.
Relationship with Dividend and Divisor: The quotient serves as a bridge between the dividend and the divisor. It establishes a direct relationship between the total quantity (dividend) and the size of each equal group (divisor). By understanding this relationship, students can recognize that the quotient represents the number of times the divisor can be subtracted from the dividend without leaving a remainder.
Estimation and Approximation: The quotient can be used as a tool for estimation and approximation. By rounding the dividend and divisor to the nearest compatible numbers, students can estimate the quotient. This strategy provides a reasonable approximation, particularly when dealing with large numbers or complex division problems.
Problem-Solving Applications: The quotient finds practical applications in various problem-solving scenarios. For example, if a baker wants to divide 36 cupcakes equally among 6 boxes, the quotient of 6 indicates that each box will contain 6 cupcakes. This understanding helps students apply division concepts to real-life situations.

In summary, the quotient plays a pivotal role in third-grade division problems. It represents the numerical answer, establishes relationships between the dividend and divisor, facilitates estimation and approximation, and finds applications in problem-solving contexts. By comprehending the concept of the quotient, students develop a strong foundation for division and enhance their mathematical problem-solving abilities.

Remainder

In the realm of third-grade division problems, the concept of the remainder plays a significant role in developing students’ understanding of the division process and its applications. The remainder represents the amount left over after the dividend has been divided evenly by the divisor. Comprehending the remainder’s significance is vital for students to grasp the nuances of division and solve problems accurately and efficiently.

The remainder arises when the dividend is not perfectly divisible by the divisor. This situation occurs when the dividend is smaller than the product of the divisor and the quotient. For instance, if we divide 13 apples equally among 4 baskets, we cannot evenly distribute all the apples. After dividing 12 apples (3 apples in each basket), we are left with 1 apple, which represents the remainder.

Understanding the remainder is important for several reasons. Firstly, it helps students recognize that not all division problems result in a whole number quotient. Secondly, it allows students to interpret remainders in real-life contexts. In the apple-basket example, the remainder of 1 apple indicates that we cannot make another full basket, and the extra apple needs to be considered separately.

Furthermore, the remainder can be used to estimate quotients and check the accuracy of division calculations. By considering the relationship between the dividend, divisor, and remainder, students can develop strategies for approximating quotients and identifying errors in their work.

In conclusion, the remainder is an integral component of third-grade division problems. It helps students understand the concept of divisibility, interpret remainders in practical situations, estimate quotients, and verify the accuracy of their division calculations. By mastering the remainder’s significance, students strengthen their problem-solving skills and develop a deeper understanding of the division process.

Division algorithm

In the realm of third-grade division problems, the division algorithm stands as a fundamental tool that empowers students with a structured and systematic approach to solving division problems. This step-by-step process enables students to break down complex division problems into smaller, manageable steps, fostering a deeper understanding of the division concept and enhancing their problem-solving abilities.

The division algorithm provides a clear roadmap for students to follow, regardless of the complexity of the division problem. By adhering to the algorithm’s steps, students can minimize errors and develop a consistent approach to solving division problems. Moreover, the algorithm helps students to identify and apply the key concepts of division, including the dividend, divisor, quotient, and remainder.

Real-life examples abound where the division algorithm proves its practical significance. Consider a scenario where a group of 24 students needs to be divided equally into 4 teams. Using the division algorithm, we can determine that each team will have 6 students, with no students left over. This demonstrates how the division algorithm can be applied to solve practical problems, making it an indispensable tool for students in their everyday lives.

In summary, the division algorithm serves as a vital component of third-grade division problems, providing students with a systematic and effective approach to solving division problems. Its importance lies in its ability to break down complex problems into manageable steps, reinforce key division concepts, and equip students with a valuable problem-solving tool for real-life applications.

Division strategies

Division strategies, particularly mental math strategies, play a pivotal role in third-grade division problems. These strategies empower students to solve division problems efficiently and accurately using their cognitive abilities, fostering a deeper understanding of the division concept and enhancing their problem-solving skills.

Mental math strategies provide students with alternative methods to solve division problems without relying solely on traditional algorithms. These strategies leverage patterns, estimation, and logical reasoning to approximate quotients and find exact answers. For instance, the “chunking” strategy involves breaking down the dividend into smaller, more manageable chunks, making the division process more accessible. Another strategy, known as “compensation,” allows students to adjust the dividend or divisor slightly to make the problem easier to solve mentally.

The significance of division strategies extends beyond the classroom, as they equip students with valuable problem-solving skills applicable to real-life situations. Consider a scenario where a group of students needs to divide a bag of 24 marbles equally among themselves. Using mental math strategies, students can quickly estimate that each student will receive approximately 6 marbles, without having to perform long division. This demonstrates how division strategies empower students to make informed decisions and solve problems efficiently in everyday life.

In conclusion, division strategies are an integral component of third-grade division problems. They provide students with alternative methods to solve division problems, foster a deeper understanding of the division concept, and enhance their problem-solving abilities. By incorporating mental math strategies into their learning, students develop valuable skills that extend beyond the classroom and empower them to tackle real-life problems with confidence.

Word problems

Word problems are an essential component of third-grade division problems, providing a bridge between abstract mathematical concepts and practical, real-world applications. By incorporating word problems into division instruction, teachers can make learning division more meaningful and engaging for students, while also developing their problem-solving skills and critical thinking abilities.

Understanding the problem: Word problems require students to read and comprehend the problem statement, identify the relevant information, and determine the mathematical operation that needs to be performed. This process develops students’ reading comprehension skills and their ability to apply mathematical concepts to real-world situations.
Applying division concepts: Word problems provide students with opportunities to apply division concepts in a meaningful context. For example, a problem about sharing a bag of marbles equally among friends requires students to understand the concept of division as sharing or partitioning into equal parts.
Developing problem-solving strategies: Word problems encourage students to develop and apply problem-solving strategies. They may need to use estimation, guess-and-check, or other strategies to find the solution. This process helps students to develop their problem-solving skills and their ability to persevere in the face of challenges.
Making connections to other subject areas: Word problems often incorporate elements from other subject areas, such as science, social studies, or language arts. This helps students to make connections between different areas of their learning and to see the relevance of mathematics in the real world.

In conclusion, word problems play a vital role in third-grade division problems. They provide students with opportunities to apply division concepts in a meaningful context, develop their problem-solving skills, and make connections to other subject areas. By incorporating word problems into instruction, teachers can make learning division more engaging and effective for students.

In the realm of elementary mathematics, third-grade division problems hold a significant place, introducing students to the fundamental concept of splitting a quantity into equal parts. These problems, often encountered in and worksheets, lay the groundwork for more advanced mathematical operations and problem-solving skills.

Division, in its essence, is the inverse operation of multiplication. It involves determining how many times a smaller number (divisor) can be subtracted from a larger number (dividend) without leaving a remainder. This concept finds practical applications in various real-world scenarios, such as distributing objects equally among a group or measuring ingredients for recipes.

Solving division problems in third grade typically involves using basic algorithms, such as the traditional long division method or the partial quotients method. These algorithms provide a step-by-step process for finding the quotient, which represents the number of equal parts, and the remainder, if any.

FAQs on “3rd Grade Division Problems”

This section addresses frequently asked questions (FAQs) related to division problems commonly encountered in third grade. These questions aim to clarify common misconceptions and provide a deeper understanding of the concept of division.

Question 1: What is the most efficient method for solving division problems?

The traditional long division method is commonly used for solving division problems. It involves a step-by-step process of dividing the dividend by the divisor, bringing down the next digit of the dividend, and repeating the process until the remainder is zero or the desired accuracy is achieved.

Question 2: How can I check the accuracy of my division answers?

One way to check the accuracy of your division answer is by multiplying the quotient by the divisor and adding the remainder (if any). The result should be equal to the original dividend. If it is not, then there may be an error in your calculation.

Question 3: What is the difference between division and multiplication?

Division and multiplication are inverse operations. Division involves finding how many times a smaller number (divisor) can be subtracted from a larger number (dividend), while multiplication involves finding the total when a number is added to itself a certain number of times.

Question 4: How can I solve division problems mentally?

Mental math strategies can be used to solve simple division problems. For example, you can use the “chunking” method, where you break down the dividend into smaller, more manageable chunks, or the “compensation” method, where you adjust the dividend or divisor slightly to make the problem easier to solve mentally.

Question 5: What are some real-world applications of division?

Division has numerous real-world applications, such as distributing objects equally among a group, measuring ingredients for recipes, calculating unit rates, and solving problems involving ratios and proportions.

Question 6: How can I improve my division skills?

Regular practice is key to improving your division skills. You can practice solving division problems using different methods, such as long division, partial quotients, or mental math strategies. Additionally, you can use online resources, worksheets, or textbooks to supplement your practice.

Summary: Division is a fundamental operation in mathematics that involves splitting a quantity into equal parts. By understanding the concept of division and practicing different methods for solving division problems, you can develop strong division skills that will benefit you in various academic and real-world situations.

Transition to the next article section: For further exploration of division problems, you can refer to the following resources…

Conclusion on “3rd Grade Division Problems”

In summary, third grade division problems serve as a critical stepping stone in students’ mathematical development. By mastering the concepts and strategies involved in division, students lay a solid foundation for more advanced mathematical operations and problem-solving skills.

Division plays a fundamental role in various real-world applications, from distributing objects equally to measuring ingredients for recipes. Equipping students with strong division skills empowers them to navigate everyday situations confidently and make informed decisions.

As students progress in their mathematical journey, division remains a cornerstone of problem-solving and critical thinking. The ability to divide numbers accurately and efficiently is essential for solving complex problems and making sense of the world around us.