Converting Mixed Numbers to Improper Fractions Worksheet
A mixed number is a number that has a whole number part and a fractional part. An improper fraction is a fraction that has a numerator that is greater than or equal to its denominator. Converting a mixed number to an improper fraction is a simple process that can be done by multiplying the whole number part by the denominator of the fractional part and then adding the numerator of the fractional part. The result is the numerator of the improper fraction, and the denominator is the same as the denominator of the fractional part.
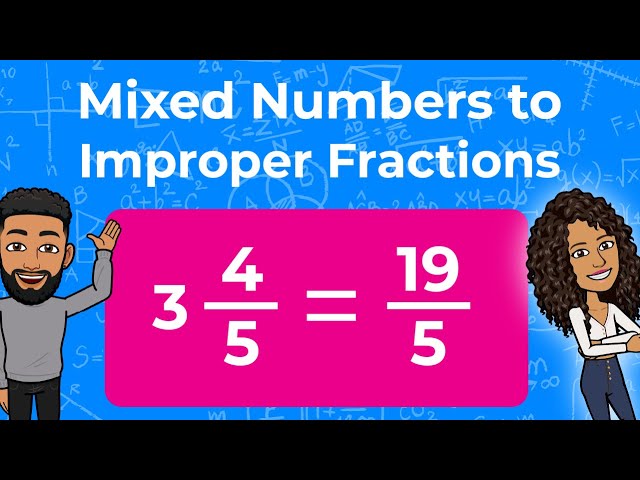
For example, to convert the mixed number $2\frac{1}{2}$ to an improper fraction, we would multiply the whole number part, 2, by the denominator of the fractional part, 2, to get 4. We would then add the numerator of the fractional part, 1, to get 5. The result, 5, is the numerator of the improper fraction, and the denominator is 2. Therefore, the improper fraction equivalent of $2\frac{1}{2}$ is $\frac{5}{2}$.
Converting mixed numbers to improper fractions can be helpful for performing certain mathematical operations, such as addition and subtraction of fractions. It can also be helpful for understanding the relationship between mixed numbers and fractions.
Steps to Create a Converting Mixed Numbers to Improper Fractions Worksheet
- Start with a table with two columns, one labeled “Mixed Number” and one labeled “Improper Fraction.”
- In the “Mixed Number” column, list the mixed numbers that you want to convert.
- In the “Improper Fraction” column, convert each mixed number to an improper fraction using the steps outlined above.
- Once you have converted all of the mixed numbers to improper fractions, you can use the worksheet to practice converting improper fractions back to mixed numbers.
Here is an example of a Converting Mixed Numbers to Improper Fractions Worksheet:
| Mixed Number | Improper Fraction |
|---|---|
| $2\frac{1}{2}$ | $\frac{5}{2}$ |
| $3\frac{1}{4}$ | $\frac{13}{4}$ |
| $4\frac{3}{5}$ | $\frac{23}{5}$ |
This worksheet can be used by students to practice converting mixed numbers to improper fractions. It can also be used by teachers to assess students’ understanding of this concept.
Converting Mixed Numbers to Improper Fractions Worksheet
Converting mixed numbers to improper fractions is a fundamental mathematical skill that involves transforming a number expressed as a combination of a whole number and a fraction into an equivalent fraction with a larger numerator than its denominator. This concept plays a crucial role in various mathematical operations and applications. Here are seven key aspects related to converting mixed numbers to improper fractions worksheet:
- Definition: Understanding the concept of mixed numbers and improper fractions.
- Method: Learning the steps involved in converting mixed numbers to improper fractions.
- Practice: Solving exercises to reinforce the conversion process.
- Applications: Recognizing the use of improper fractions in mathematical operations like addition, subtraction, and multiplication.
- Fractions: Exploring the relationship between mixed numbers and fractions.
- Worksheet: Utilizing a structured worksheet to guide the learning process.
- Assessment: Evaluating understanding through worksheet exercises.
These aspects collectively provide a comprehensive framework for understanding the topic of converting mixed numbers to improper fractions. Worksheets serve as valuable tools for practicing and mastering this skill, fostering a deeper comprehension of the concept and its applications in mathematical operations.
Definition
Understanding the concept of mixed numbers and improper fractions is a fundamental prerequisite for successfully converting mixed numbers to improper fractions using a worksheet. A mixed number is a number that combines a whole number and a fraction, such as $2\frac{1}{2}$. An improper fraction is a fraction where the numerator is greater than or equal to the denominator, such as $\frac{5}{2}$. Converting a mixed number to an improper fraction involves multiplying the whole number by the denominator of the fraction and then adding the numerator of the fraction. The result is the numerator of the improper fraction, and the denominator remains the same as the denominator of the original fraction.
-
Components: Mixed numbers consist of a whole number part and a fractional part, while improper fractions have a numerator and a denominator.
In the mixed number $2\frac{1}{2}$, 2 is the whole number part and $\frac{1}{2}$ is the fractional part. In the improper fraction $\frac{5}{2}$, 5 is the numerator and 2 is the denominator.
-
Examples: Mixed numbers can be encountered in everyday situations, such as measuring ingredients in a recipe or calculating distances on a map. Improper fractions are commonly used in mathematical operations, such as addition and subtraction of fractions.
For example, in a recipe that calls for $2\frac{1}{2}$ cups of flour, the mixed number represents the total amount of flour needed. In a math problem involving the addition of fractions, such as $\frac{1}{2} + \frac{3}{4}$, the improper fraction $\frac{5}{4}$ is used as an intermediate step to simplify the calculation.
-
Implications for converting mixed numbers to improper fractions worksheet: A clear understanding of the concept of mixed numbers and improper fractions is essential for students to successfully complete a converting mixed numbers to improper fractions worksheet. The worksheet typically provides exercises where students are given mixed numbers and asked to convert them to improper fractions.
Without a solid grasp of the underlying concepts, students may struggle to apply the correct steps and arrive at the correct answers. Therefore, it is important for students to have a strong foundation in understanding mixed numbers and improper fractions before attempting a worksheet on this topic.
In summary, the definition and understanding of mixed numbers and improper fractions lay the groundwork for students to effectively engage with a converting mixed numbers to improper fractions worksheet. By comprehending the components, examples, and implications of these concepts, students can approach the exercises with confidence and develop proficiency in this mathematical skill.
Method
Understanding the method involved in converting mixed numbers to improper fractions is crucial for successful completion of a converting mixed numbers to improper fractions worksheet. The method consists of a series of steps that, when followed correctly, result in the correct improper fraction equivalent of the mixed number.
-
Step 1: Multiply the whole number by the denominator of the fraction.
This step involves multiplying the whole number part of the mixed number by the denominator of the fractional part. The result of this multiplication is added to the numerator of the fractional part in the next step.
-
Step 2: Add the numerator of the fraction to the product obtained in Step 1.
The numerator of the fractional part of the mixed number is added to the result obtained in Step 1. This sum becomes the numerator of the improper fraction.
-
Step 3: The denominator of the improper fraction is the same as the denominator of the original fraction.
The denominator of the improper fraction remains the same as the denominator of the fractional part of the mixed number.
By following these steps, students can systematically convert any mixed number to its equivalent improper fraction. The converting mixed numbers to improper fractions worksheet provides ample opportunities for students to practice this method and develop proficiency in converting mixed numbers to improper fractions.
Practice
Practice plays a pivotal role in solidifying the understanding of converting mixed numbers to improper fractions. A converting mixed numbers to improper fractions worksheet provides ample opportunities for students to engage in exercises that reinforce the conversion process, leading to a deeper comprehension of the concept and its applications.
-
Facet 1: Reinforcing the Steps
By repeatedly solving exercises, students solidify their understanding of the individual steps involved in converting mixed numbers to improper fractions. Each exercise requires them to apply the method, reinforcing their grasp of the process and building automaticity.
-
Facet 2: Identifying Patterns
As students work through numerous exercises, they begin to recognize patterns and relationships between mixed numbers and improper fractions. This enhanced understanding enables them to approach similar problems with greater confidence and efficiency.
-
Facet 3: Developing Fluency
Consistent practice through a converting mixed numbers to improper fractions worksheet enhances students’ fluency in converting mixed numbers to improper fractions. With repeated exposure, the process becomes more familiar and less daunting, freeing up cognitive resources for problem-solving and higher-order thinking.
-
Facet 4: Building Confidence
Solving exercises and experiencing success in converting mixed numbers to improper fractions boosts students’ confidence in their mathematical abilities. This positive reinforcement motivates them to tackle more challenging problems and persevere in their mathematical journey.
In conclusion, the connection between “Practice: Solving exercises to reinforce the conversion process” and “converting mixed numbers to improper fractions worksheet” is crucial. By engaging in practice exercises, students not only reinforce the conversion process but also develop a deeper understanding of the concept, enhance their problem-solving skills, and build confidence in their mathematical abilities.
Applications
The connection between “Applications: Recognizing the use of improper fractions in mathematical operations like addition, subtraction, and multiplication” and “converting mixed numbers to improper fractions worksheet” lies in the practical significance of converting mixed numbers to improper fractions. Improper fractions are not only mathematical entities but also essential tools for performing various mathematical operations. A converting mixed numbers to improper fractions worksheet provides a platform for students to develop an understanding of these applications and their importance in mathematical problem-solving.
-
Facet 1: Addition and Subtraction of Fractions
Improper fractions are particularly useful when adding or subtracting fractions. By converting mixed numbers to improper fractions, students can find a common denominator and perform the operations more efficiently. For instance, to add $2\frac{1}{2}$ and $3\frac{1}{4}$, converting them to improper fractions ($\frac{5}{2}$ and $\frac{13}{4}$) allows for the calculation of $\frac{5}{2} + \frac{13}{4} = \frac{26}{4} + \frac{13}{4} = \frac{39}{4}$, which can then be converted back to a mixed number ($9\frac{3}{4}$).
-
Facet 2: Multiplication of Fractions
Multiplication of fractions involves multiplying both the numerators and the denominators. When multiplying mixed numbers, converting them to improper fractions simplifies the process. For example, to multiply $2\frac{1}{2}$ by $3\frac{1}{4}$, converting them to improper fractions ($\frac{5}{2}$ and $\frac{13}{4}$) allows for easier multiplication, resulting in $\frac{5}{2} \times \frac{13}{4} = \frac{65}{8}$, which can be further simplified to an improper fraction or converted back to a mixed number ($8\frac{1}{8}$).
-
Facet 3: Real-Life Applications
Converting mixed numbers to improper fractions has practical applications beyond theoretical mathematics. In cooking, recipes often involve ingredients measured in mixed numbers. Converting these measurements to improper fractions ensures accurate scaling of ingredients, leading to successful culinary outcomes. Similarly, in carpentry and other construction-related fields, improper fractions are used to represent measurements and dimensions, enabling precise calculations and accurate construction.
By integrating these applications into a converting mixed numbers to improper fractions worksheet, students can appreciate the practical significance of this conversion. It fosters a deeper understanding of mathematical operations and their real-world implications, making the learning process more meaningful and engaging.
Fractions
In mathematics, fractions and mixed numbers are two closely related concepts. A fraction represents a part of a whole, while a mixed number is a combination of a whole number and a fraction. Converting mixed numbers to improper fractions is a fundamental skill that involves understanding the relationship between these two representations of numbers.
-
Facet 1: Understanding the Components
Fractions consist of a numerator and a denominator, while mixed numbers have a whole number part and a fractional part. To convert a mixed number to an improper fraction, the whole number part is multiplied by the denominator of the fractional part, and the result is added to the numerator of the fractional part. The denominator of the improper fraction remains the same as the denominator of the fractional part of the mixed number.
-
Facet 2: Recognizing Equivalence
Mixed numbers and improper fractions can represent the same value. For example, the mixed number $2\frac{1}{2}$ is equivalent to the improper fraction $\frac{5}{2}$. Converting mixed numbers to improper fractions allows for easier comparison and manipulation of these numbers.
-
Facet 3: Simplifying Calculations
Improper fractions are often used in mathematical operations, such as addition, subtraction, and multiplication. Converting mixed numbers to improper fractions can simplify these calculations. For instance, adding the mixed numbers $2\frac{1}{2}$ and $3\frac{1}{4}$ requires converting them to improper fractions ($\frac{5}{2}$ and $\frac{13}{4}$) to find a common denominator and perform the operation.
-
Facet 4: Applications in Real-World Contexts
Mixed numbers and improper fractions are used in various real-world applications. For example, in cooking, recipes often use mixed numbers to specify ingredient quantities. Converting these mixed numbers to improper fractions ensures accurate scaling of ingredients, leading to successful culinary outcomes.
In summary, understanding the relationship between mixed numbers and fractions is crucial for converting mixed numbers to improper fractions. A converting mixed numbers to improper fractions worksheet provides opportunities for students to explore this relationship, develop their conversion skills, and apply these concepts in practical contexts.
Worksheet
A converting mixed numbers to improper fractions worksheet serves as a valuable tool in guiding students through the learning process. It provides a structured framework for practicing and mastering the conversion of mixed numbers to improper fractions, reinforcing understanding and enhancing problem-solving skills.
-
Facet 1: Step-by-Step Guidance
A well-designed converting mixed numbers to improper fractions worksheet presents clear instructions and examples, guiding students through each step of the conversion process. This structured approach ensures that students develop a systematic understanding of the method and can apply it consistently.
-
Facet 2: Varied Practice Exercises
A comprehensive converting mixed numbers to improper fractions worksheet includes a range of practice exercises that cover different levels of difficulty. This variety challenges students, helps them identify areas for improvement, and fosters a deeper understanding of the concept.
-
Facet 3: Immediate Feedback
Many converting mixed numbers to improper fractions worksheets provide answer keys or online platforms that offer immediate feedback on student responses. This allows students to check their work, identify errors, and make corrections, reinforcing their learning and promoting self-assessment.
-
Facet 4: Independent Learning
Converting mixed numbers to improper fractions worksheets can facilitate independent learning, allowing students to practice and improve their skills outside of the classroom setting. They can work at their own pace, review concepts as needed, and reinforce their understanding through repeated practice.
In summary, a converting mixed numbers to improper fractions worksheet provides a structured and guided learning experience that supports students in developing a strong foundation in this mathematical concept. It offers step-by-step guidance, varied practice opportunities, immediate feedback, and encourages independent learning, contributing to a deeper understanding and proficiency in converting mixed numbers to improper fractions.
Assessment
Assessment plays a crucial role in the learning process, providing valuable insights into students’ understanding of converting mixed numbers to improper fractions. A converting mixed numbers to improper fractions worksheet serves as an effective assessment tool, enabling educators to evaluate students’ proficiency in this mathematical concept.
-
Facet 1: Monitoring Progress
Worksheet exercises allow teachers to monitor students’ progress in real-time. By observing students’ responses, educators can identify areas where students excel and areas that require additional support. This ongoing assessment helps tailor instruction to meet individual student needs and ensures that all students are making meaningful progress.
-
Facet 2: Identifying Common Errors
Worksheet exercises reveal common errors that students make when converting mixed numbers to improper fractions. By analyzing students’ responses, teachers can pinpoint specific misconceptions or areas of difficulty. This information helps educators address these errors through targeted interventions, strengthening students’ understanding.
-
Facet 3: Providing Feedback
Worksheet exercises provide opportunities for formative feedback. Teachers can offer personalized feedback on students’ work, highlighting areas of strength and providing guidance for improvement. This feedback loop helps students identify their strengths and weaknesses, promoting self-reflection and fostering a growth mindset.
-
Facet 4: Preparing for Assessments
Worksheet exercises serve as valuable preparation for summative assessments, such as tests or exams. By practicing the conversion process repeatedly, students become more confident in their abilities and develop the skills necessary to perform well on formal assessments.
In conclusion, assessment is an integral part of the learning process, and a converting mixed numbers to improper fractions worksheet provides a valuable tool for evaluating students’ understanding. Through ongoing monitoring, identification of errors, provision of feedback, and preparation for assessments, this worksheet supports students in developing proficiency in converting mixed numbers to improper fractions.
A converting mixed numbers to improper fractions worksheet is an educational tool designed to help students understand and practice the mathematical concept of converting mixed numbers to improper fractions. Mixed numbers are numbers that combine a whole number and a fraction, while improper fractions are fractions where the numerator is greater than or equal to the denominator. Converting mixed numbers to improper fractions is a fundamental skill in mathematics, as it allows for easier computation and problem-solving.
This worksheet provides a structured approach to learning this conversion process. It typically includes clear instructions, examples, and a variety of exercises for students to complete. By engaging with this worksheet, students can develop a deeper understanding of the relationship between mixed numbers and improper fractions, enhance their problem-solving abilities, and improve their overall mathematical proficiency.
Converting mixed numbers to improper fractions has practical applications in various fields, including cooking, carpentry, and engineering. By mastering this concept, students can confidently tackle real-world problems that require the conversion of mixed numbers to improper fractions. Furthermore, this skill forms a solid foundation for more advanced mathematical concepts, such as adding, subtracting, multiplying, and dividing fractions.
FAQs on Converting Mixed Numbers to Improper Fractions Worksheet
This section addresses frequently asked questions and misconceptions related to converting mixed numbers to improper fractions. Each question is answered concisely to provide clarity and reinforce understanding.
Question 1: What is the purpose of a converting mixed numbers to improper fractions worksheet?
Answer: A converting mixed numbers to improper fractions worksheet is designed to help students practice and master the conversion of mixed numbers to improper fractions. It provides a structured approach to learning this concept, enhancing problem-solving abilities and overall mathematical proficiency.
Question 2: How can I use this worksheet effectively?
Answer: To effectively use a converting mixed numbers to improper fractions worksheet, begin by reviewing the provided instructions and examples. Carefully work through each exercise, ensuring that you understand the steps involved. Check your answers using the answer key or online platforms to identify areas for improvement. Repeated practice is crucial for developing fluency and confidence.
Question 3: Why is it important to convert mixed numbers to improper fractions?
Answer: Converting mixed numbers to improper fractions simplifies mathematical operations such as addition, subtraction, multiplication, and division of fractions. It allows for a common denominator, making these operations more manageable and efficient.
Question 4: Are there any real-world applications of converting mixed numbers to improper fractions?
Answer: Yes, converting mixed numbers to improper fractions has practical applications in various fields. For example, in cooking, recipes often use mixed numbers to specify ingredient quantities. Converting these mixed numbers to improper fractions ensures accurate scaling and successful culinary outcomes.
Question 5: What are some common errors students make when converting mixed numbers to improper fractions?
Answer: Common errors include forgetting to multiply the whole number by the denominator, incorrectly adding the whole number and numerator, or using the improper fraction as a mixed number in subsequent calculations.
Question 6: How can I improve my accuracy and speed in converting mixed numbers to improper fractions?
Answer: Consistent practice is key to improving accuracy and speed. Utilize the converting mixed numbers to improper fractions worksheet regularly, time yourself to track your progress, and seek support from your teacher or a tutor if needed. Remember, with dedication and effort, you can develop proficiency in this mathematical skill.
In summary, a converting mixed numbers to improper fractions worksheet is a valuable tool for students to enhance their understanding of this concept and develop their mathematical abilities. By addressing common questions and misconceptions, this FAQ section provides additional support and guidance for effective learning.
Transition to the next article section: This section has provided comprehensive answers to frequently asked questions on converting mixed numbers to improper fractions. In the next section, we will delve into the benefits of using a converting mixed numbers to improper fractions worksheet, exploring how it contributes to a deeper understanding of mathematical concepts and problem-solving skills.
Conclusion
In conclusion, a converting mixed numbers to improper fractions worksheet serves as a valuable pedagogical tool that enhances students’ understanding of this mathematical concept and fosters their problem-solving abilities. Through structured practice and guided exercises, students develop a strong foundation in converting mixed numbers to improper fractions, which is essential for success in various mathematical operations and real-world applications.
The benefits of using a converting mixed numbers to improper fractions worksheet extend beyond the classroom, as this skill finds practical applications in diverse fields such as cooking, carpentry, and engineering. By mastering this concept, students gain the confidence and competence to tackle more complex mathematical challenges and contribute meaningfully to society. As educators, we must continue emphasizing the importance of this skill and providing students with ample opportunities to practice and refine it.
Youtube Video: