Elementary Math: A Comprehensive Guide to Adding and Subtracting Polynomials

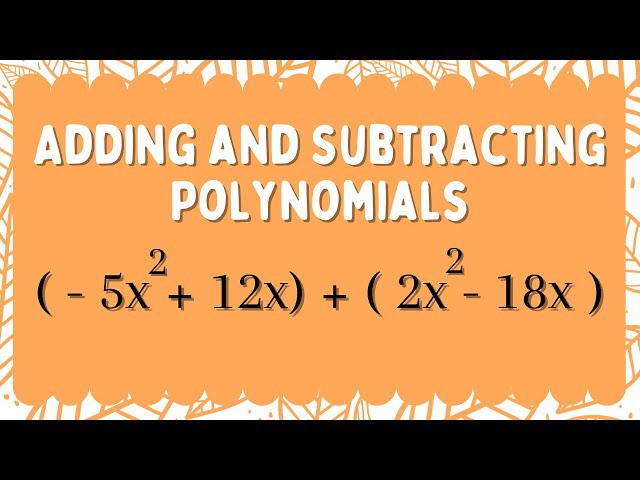
Adding and Subtracting Polynomials Worksheet

A polynomial is a mathematical expression that consists of variables and coefficients, and the operations of addition, subtraction, multiplication, and division. Adding and subtracting polynomials is a fundamental skill in algebra, and it is used in a variety of applications, such as finding the area of a triangle or solving equations.

There are a few different ways to add and subtract polynomials. One way is to use the distributive property. The distributive property states that the product of a number and a sum is equal to the sum of the products of the number and each of the addends. For example, 3(x + y) = 3x + 3y.

Another way to add and subtract polynomials is to use the FOIL method. FOIL stands for First, Outer, Inner, Last. The FOIL method is used to multiply two binomials. For example, (x + y)(x – y) = x^2 – y^2.

Once you have multiplied the two binomials, you can add or subtract the like terms. Like terms are terms that have the same variable and exponent. For example, 3x and 5x are like terms.

Here are some examples of adding and subtracting polynomials:

• (x + y) + (x – y) = 2x
• (x + y) – (x – y) = 2y
• (x + y)(x – y) = x^2 – y^2

Adding and subtracting polynomials is a relatively simple skill, but it is important to practice in order to become proficient. There are a number of online resources that can help you practice adding and subtracting polynomials, such as Khan Academy and Mathway.

Benefits of practicing adding and subtracting polynomials include:

• Improved problem-solving skills
• Increased understanding of algebra
• Better preparation for higher-level math courses

If you are struggling to add and subtract polynomials, don’t be afraid to ask for help. Your teacher or a tutor can help you understand the concepts and practice the skills.

Essential Aspects of Adding and Subtracting Polynomials

Adding and subtracting polynomials is a fundamental skill in algebra. It is used in a variety of applications, such as finding the area of a triangle or solving equations. There are a few different ways to add and subtract polynomials, and it is important to understand the essential aspects of each method in order to be successful.

• Variables: Polynomials are made up of variables, which represent unknown values.
• Coefficients: Polynomials also have coefficients, which are the numbers that are multiplied by the variables.
• Terms: Polynomials are made up of terms, which are the individual parts of the polynomial.
• Like terms: Like terms are terms that have the same variable and exponent.
• Distributive property: The distributive property is used to multiply a polynomial by a monomial.
• FOIL method: The FOIL method is used to multiply two binomials.
• Adding polynomials: To add polynomials, you add the like terms.
• Subtracting polynomials: To subtract polynomials, you subtract the like terms.

These are just a few of the essential aspects of adding and subtracting polynomials. By understanding these concepts, you will be able to add and subtract polynomials with ease.

Variables

Variables are an essential part of polynomials. They represent unknown values, which can be anything from the length of a side of a triangle to the speed of a car. When we add or subtract polynomials, we are essentially combining or separating these unknown values.

For example, let’s say we have two polynomials: 3x + 5 and 2x – 1. The variable x represents the unknown value in both polynomials. When we add these two polynomials, we get 5x + 4. This new polynomial represents the combined value of the two original polynomials.

Variables are also important for understanding the relationship between different polynomials. For example, if we have two polynomials with the same variable, we can use the distributive property to multiply them. This can be helpful for simplifying complex polynomials or for finding the area of a triangle.

Overall, variables are an essential part of polynomials. They allow us to represent unknown values and to combine or separate these values as needed. When we understand the role of variables in polynomials, we can add and subtract polynomials with ease.

Coefficients

Coefficients are an essential part of polynomials. They represent the numerical value of each term in the polynomial. When we add or subtract polynomials, we must take into account the coefficients of each term.

• The coefficient of a term determines its sign. A positive coefficient indicates that the term is positive, while a negative coefficient indicates that the term is negative. For example, in the polynomial 3x + 5, the coefficient of the x-term is 3, which means that the x-term is positive. The coefficient of the constant term is 5, which means that the constant term is positive.
• The coefficient of a term also determines its magnitude. The larger the coefficient, the greater the magnitude of the term. For example, in the polynomial 3x + 5, the coefficient of the x-term is 3, which means that the x-term has a greater magnitude than the constant term, which has a coefficient of 5.
• The coefficients of like terms must be added or subtracted when adding or subtracting polynomials. Like terms are terms that have the same variable and exponent. For example, in the polynomial 3x + 5, the x-term and the constant term are like terms. When we add these two terms, we add their coefficients, which gives us 3 + 5 = 8. The result is the coefficient of the x-term in the new polynomial.

Coefficients are an essential part of polynomials, and they play an important role in adding and subtracting polynomials. By understanding the role of coefficients, we can add and subtract polynomials with ease.

Terms

Terms are the building blocks of polynomials. They are the individual parts that are added or subtracted to create a polynomial. Each term consists of a coefficient and a variable. The coefficient is the numerical value of the term, and the variable is the unknown value. For example, in the polynomial 3x + 5, the term 3x has a coefficient of 3 and a variable of x, and the term 5 has a coefficient of 5 and a variable of 1.

When adding or subtracting polynomials, it is important to understand the concept of like terms. Like terms are terms that have the same variable and exponent. For example, in the polynomial 3x + 5, the terms 3x and 5 are like terms because they both have the variable x and the exponent 1. When adding or subtracting like terms, we simply add or subtract their coefficients. For example, 3x + 5 + 2x – 1 = 5x + 4.

• Combining like terms: When adding or subtracting polynomials, we can combine like terms to simplify the polynomial. For example, in the polynomial 3x + 5 + 2x – 1, we can combine the like terms 3x and 2x to get 5x. This simplifies the polynomial to 5x + 4.
• Factoring polynomials: We can also use terms to factor polynomials. Factoring is the process of writing a polynomial as a product of two or more polynomials. For example, the polynomial x^2 – 4 can be factored as (x + 2)(x – 2). This is because x^2 – 4 = x^2 – 2^2 = (x + 2)(x – 2).
• Solving equations: We can also use terms to solve equations. For example, to solve the equation x^2 – 4 = 0, we can factor the polynomial as (x + 2)(x – 2) = 0. This means that either x + 2 = 0 or x – 2 = 0. Solving these two equations gives us x = -2 or x = 2.

Terms are an essential part of polynomials, and they play an important role in adding, subtracting, and factoring polynomials. By understanding the concept of terms, we can work with polynomials more effectively.

Like Terms

Like terms are an essential part of adding and subtracting polynomials. When we add or subtract polynomials, we must first identify the like terms. Once we have identified the like terms, we can then add or subtract their coefficients. For example, let’s say we have the polynomial 3x + 5 + 2x – 1. The like terms in this polynomial are 3x and 2x. We can add these two terms to get 5x. The new polynomial is 5x + 5.

Like terms are also important for factoring polynomials. Factoring is the process of writing a polynomial as a product of two or more polynomials. For example, the polynomial x^2 – 4 can be factored as (x + 2)(x – 2). This is because x^2 – 4 = x^2 – 2^2 = (x + 2)(x – 2). In order to factor a polynomial, we must first identify the like terms. Once we have identified the like terms, we can then group them together and factor them out.

Understanding like terms is essential for adding, subtracting, and factoring polynomials. Without a clear understanding of like terms, it would be impossible to perform these operations.

Distributive Property

The distributive property is a fundamental property of multiplication that states that the product of a number and a sum is equal to the sum of the products of the number and each of the addends. In other words, for any number $a$, and any numbers $b$ and $c$, we have $$a(b + c) = ab + ac.$$

The distributive property is used extensively in mathematics, including in the context of adding and subtracting polynomials.

• Multiplying a polynomial by a monomial: The distributive property can be used to multiply a polynomial by a monomial. For example, to multiply the polynomial $3x + 5$ by the monomial $2$, we can use the distributive property as follows: $$(3x + 5)2 = 3x(2) + 5(2) = 6x + 10.$$
• Adding and subtracting polynomials: The distributive property can also be used to add and subtract polynomials. For example, to add the polynomials $3x + 5$ and $2x – 1$, we can use the distributive property as follows: $$(3x + 5) + (2x – 1) = 3x + 5 + 2x – 1 = 5x + 4.$$

The distributive property is an essential tool for adding, subtracting, and multiplying polynomials. By understanding the distributive property, students can develop a deeper understanding of polynomial operations.

FOIL Method

The FOIL method is a technique used to multiply two binomials. It is a useful tool for simplifying polynomial expressions and for solving equations. The FOIL method is an acronym that stands for First, Outer, Inner, Last. These four steps refer to the four pairs of terms that are multiplied when multiplying two binomials.

• First: Multiply the first terms of each binomial.
• Outer: Multiply the outer terms of each binomial.
• Inner: Multiply the inner terms of each binomial.
• Last: Multiply the last terms of each binomial.

For example, let’s multiply the binomials (x + 2) and (x – 3) using the FOIL method:

• First: (x)(x) = x^2
• Outer: (x)(-3) = -3x
• Inner: (2)(x) = 2x
• Last: (2)(-3) = -6

Adding these four products together, we get x^2 – x – 6. Therefore, (x + 2)(x – 3) = x^2 – x – 6.

The FOIL method can be used to multiply any two binomials. It is a quick and easy way to simplify polynomial expressions and to solve equations.

Adding polynomials

Adding polynomials is a fundamental operation in algebra. It is used to combine like terms, simplify expressions, and solve equations. To add polynomials, you add the coefficients of like terms. Like terms are terms that have the same variable and exponent. For example, in the polynomial 3x + 5 + 2x – 1, the like terms are 3x and 2x. We can add these terms to get 5x. The new polynomial is 5x + 5.

Adding subtracting polynomials worksheet is a resource that can help students practice adding polynomials. The worksheet typically includes a variety of problems, ranging from simple to complex. By completing the worksheet, students can develop a better understanding of how to add polynomials and how to apply this skill to real-world problems.

Adding polynomials is an important skill for students to master. It is a foundational skill that is used in many different areas of mathematics, including algebra, geometry, and calculus. By understanding how to add polynomials, students can develop a stronger foundation in mathematics and be better prepared for success in future math courses.

Subtracting Polynomials

Subtracting polynomials is the inverse operation of adding polynomials. To subtract polynomials, you subtract the coefficients of like terms. Like terms are terms that have the same variable and exponent. For example, in the polynomial 3x + 5 + 2x – 1, the like terms are 3x and 2x. We can subtract these terms to get x. The new polynomial is x + 5.

Subtracting polynomials is a useful skill for students to master. It is used to simplify expressions, solve equations, and find the area of geometric shapes. By understanding how to subtract polynomials, students can develop a stronger foundation in mathematics and be better prepared for success in future math courses.

• Components of Subtracting Polynomials: Subtracting polynomials involves identifying like terms and subtracting their coefficients. This process requires careful attention to the signs of the coefficients, as well as the variables and exponents involved.
• Examples in Real Life: Subtracting polynomials has practical applications in various fields. For instance, in physics, it is used to calculate the net force acting on an object by subtracting the opposing forces. In economics, it is employed to determine the profit or loss by subtracting expenses from revenue.
• Implications in “Adding Subtracting Polynomials Worksheet”: Worksheets on adding and subtracting polynomials provide structured practice for students to develop proficiency in these operations. By completing such worksheets, students can reinforce their understanding of like terms and apply subtraction techniques to simplify expressions.
• Additional Insights: Subtracting polynomials is closely related to adding polynomials, and both operations are essential for manipulating algebraic expressions. By understanding the relationship between addition and subtraction of polynomials, students can develop a deeper comprehension of polynomial operations.

In summary, subtracting polynomials is a fundamental operation in algebra with various applications across disciplines. The “adding subtracting polynomials worksheet” provides a valuable tool for students to practice and master this skill, laying the groundwork for success in more advanced mathematics.

An “adding subtracting polynomials worksheet” is a valuable educational tool designed to provide practice and reinforce understanding of polynomial operations, particularly adding and subtracting polynomials. These worksheets typically consist of a series of problems or exercises that require students to apply their knowledge of polynomial operations to simplify expressions or solve equations.

Polynomials are algebraic expressions consisting of variables, coefficients, and exponents. Adding and subtracting polynomials involves combining or separating like terms, which are terms with the same variable and exponent. Worksheets on this topic help students develop proficiency in identifying like terms and performing the appropriate operations, ensuring a solid foundation in polynomial algebra.

The benefits of using adding subtracting polynomials worksheets extend beyond the classroom. Polynomial operations are essential in various fields, including physics, engineering, and economics. By practicing these operations, students not only enhance their mathematical abilities but also develop critical thinking and problem-solving skills.

In summary, adding subtracting polynomials worksheets provide an effective means for students to practice and master polynomial operations. These worksheets contribute to a deeper understanding of algebraic concepts and prepare students for more advanced mathematics and real-world applications.

FAQs about Adding and Subtracting Polynomials

Question 1: What is a polynomial?

Answer: A polynomial is an algebraic expression consisting of variables, coefficients, and exponents. Variables represent unknown values, coefficients are numerical values, and exponents indicate the power to which the variable is raised.

Question 2: What does it mean to add or subtract polynomials?

Answer: Adding or subtracting polynomials involves combining or separating like terms, which are terms with the same variable and exponent. When adding like terms, their coefficients are added or subtracted, while keeping the same variable and exponent.

Question 3: Why is it important to practice adding and subtracting polynomials?

Answer: Practicing these operations helps develop proficiency in polynomial algebra, which is essential for solving equations, simplifying expressions, and understanding more advanced mathematical concepts.

Question 4: Are there any tricks or shortcuts for adding and subtracting polynomials?

Answer: While there are no specific tricks, understanding the concept of like terms and grouping similar terms can simplify the process.

Question 5: How can I check my answers when adding or subtracting polynomials?

Answer: To verify your results, substitute the original values of the variables into the simplified polynomial and ensure that the equation holds true.

Question 6: What are some real-world applications of adding and subtracting polynomials?

Answer: Polynomial operations find applications in fields such as physics, engineering, and economics, where they are used to model various phenomena and solve problems.

Summary: Adding and subtracting polynomials are fundamental mathematical operations that involve combining or separating like terms. Practicing these operations through worksheets or exercises strengthens algebraic skills and prepares individuals for more complex mathematical concepts and real-world applications.

Transition: To enhance your understanding of adding and subtracting polynomials, consider exploring additional resources, such as online tutorials or textbooks that provide detailed explanations and examples.

Conclusion

In this article, we explored the concept of adding and subtracting polynomials, emphasizing the importance of understanding like terms and applying the appropriate operations. The “adding subtracting polynomials worksheet” was introduced as a valuable tool for practicing these operations and developing proficiency in polynomial algebra.

Mastering polynomial operations is crucial for success in more advanced mathematics and various real-world applications. By utilizing worksheets and other resources to practice and reinforce these concepts, students and individuals can enhance their mathematical abilities, critical thinking skills, and problem-solving capabilities.

Youtube Video: