Easy-to-Master Adding Fractions with Different Denominators Worksheet

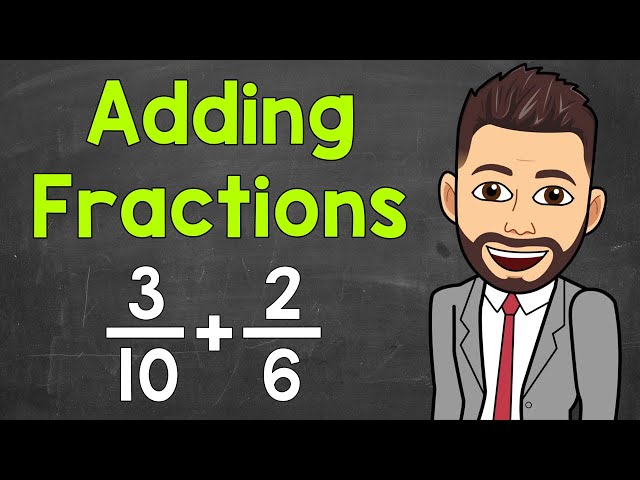
Adding Fractions with Different Denominator Worksheet

Adding fractions with different denominators can be a challenge for students. A fraction chart or diagram can be a helpful tool for students to visualize the process and understand the steps involved. There are a few different ways to create a fraction chart or diagram. One common method is to use a T-chart.

Steps to create a fraction chart or diagram:

Draw a T-chart with two columns. Label the first column “Numerator” and the second column “Denominator.”
In the first column, list the numerators of the fractions you are adding.
In the second column, list the denominators of the fractions you are adding.
Multiply the numerator of each fraction by the denominator of the other fraction.
Add the products of the numerators.
Add the denominators of the fractions.
Simplify the fraction, if possible.

Benefits of using a fraction chart or diagram:

Helps students visualize the process of adding fractions with different denominators.
Makes it easier for students to understand the steps involved in the process.
Can be used as a reference tool for students who need help with adding fractions.

Fraction charts or diagrams are a valuable tool for students who are learning to add fractions with different denominators. They can help students to understand the process and visualize the steps involved. With practice, students can become proficient at adding fractions with different denominators using a fraction chart or diagram.

Exploring Key Aspects of Adding Fractions with Different Denominator Worksheet

Adding fractions with different denominators is a fundamental skill in mathematics. A worksheet on this topic can help students practice and master this skill. Here are eight key aspects of adding fractions with different denominator worksheet:

Numerator: The top number of a fraction.
Denominator: The bottom number of a fraction.
Equivalent fractions: Fractions that have the same value, even if they look different.
Common denominator: A denominator that is the same for all the fractions being added.
Least common multiple (LCM): The smallest number that is a multiple of all the denominators.
Cross-multiplication: A method for finding equivalent fractions with a common denominator.
Simplifying fractions: Reducing fractions to their simplest form.
Adding fractions with different denominators: The process of combining fractions with different denominators into a single fraction.

These key aspects are all essential for understanding how to add fractions with different denominators. By practicing with a worksheet, students can develop a strong foundation in this important skill.

Numerator

In the context of adding fractions with different denominators, the numerator plays a crucial role in determining the value of the fraction. The numerator represents the number of parts of the whole that are being considered. For instance, in the fraction 3/4, the numerator 3 indicates that three parts of the whole are being considered. Understanding the numerator is essential for accurately adding fractions with different denominators.

Identifying the numerator: The numerator is the top number of a fraction. It represents the number of parts of the whole that are being considered.
Comparing numerators: When adding fractions with different denominators, it is important to compare the numerators to determine which fraction has a larger value.
Adding numerators: Once the fractions have been converted to equivalent fractions with a common denominator, the numerators can be added together to find the numerator of the sum.

By understanding the role of the numerator in adding fractions with different denominators, students can develop a strong foundation in this important skill. Worksheets that focus on adding fractions with different denominators can help students practice identifying, comparing, and adding numerators to solve problems accurately.

Denominator

The denominator plays a crucial role in adding fractions with different denominators. It represents the total number of parts in the whole being considered. For instance, in the fraction 3/4, the denominator 4 indicates that the whole is divided into four equal parts. Understanding the denominator is essential for accurately adding fractions with different denominators.

Identifying the denominator: The denominator is the bottom number of a fraction. It represents the total number of parts in the whole being considered.
Comparing denominators: When adding fractions with different denominators, it is important to compare the denominators to determine which fraction has a smaller part of the whole.
Finding a common denominator: To add fractions with different denominators, it is necessary to find a common denominator, which is a multiple of all the denominators.
Converting fractions to equivalent fractions: Once a common denominator has been found, the fractions can be converted to equivalent fractions with the common denominator.

By understanding the role of the denominator in adding fractions with different denominators, students can develop a strong foundation in this important skill. Worksheets that focus on adding fractions with different denominators can help students practice identifying, comparing, and converting denominators to solve problems accurately.

Equivalent fractions

When adding fractions with different denominators, it is often necessary to convert the fractions to equivalent fractions with a common denominator. This is because the denominators represent the number of parts in the whole, and in order to add fractions, the wholes must be the same. For example, the fractions 1/2 and 2/4 are equivalent because they both represent one half of the whole.

Converting fractions to equivalent fractions: When adding fractions with different denominators, the first step is to convert the fractions to equivalent fractions with a common denominator. This can be done by multiplying the numerator and denominator of each fraction by the same number.
Finding a common denominator: The common denominator is the smallest number that is a multiple of all the denominators of the fractions being added. For example, the common denominator of 1/2 and 2/4 is 4.
Adding fractions with equivalent fractions: Once the fractions have been converted to equivalent fractions with a common denominator, they can be added by adding the numerators and keeping the common denominator.

Understanding equivalent fractions is essential for adding fractions with different denominators. By practicing with worksheets that focus on adding fractions with different denominators, students can develop a strong foundation in this important skill.

Common denominator

When adding fractions with different denominators, it is necessary to find a common denominator. This is because the denominators represent the number of parts in the whole, and in order to add fractions, the wholes must be the same. For example, to add the fractions 1/2 and 1/4, we need to find a common denominator. The smallest number that is a multiple of both 2 and 4 is 4, so 4 is the common denominator. We can then convert the fractions 1/2 and 1/4 to equivalent fractions with a denominator of 4: 1/2 = 2/4 and 1/4 = 1/4. Now that the fractions have the same denominator, we can add them: 2/4 + 1/4 = 3/4.

Finding a common denominator is an essential step when adding fractions with different denominators. Without a common denominator, it is not possible to add the fractions because the wholes are different. Worksheets that focus on adding fractions with different denominators can help students practice finding common denominators and adding fractions with different denominators.

In real-life applications, adding fractions with different denominators is a common task. For example, a baker may need to add fractions to calculate the total amount of ingredients needed for a recipe. A construction worker may need to add fractions to calculate the total length of materials needed for a project. By understanding how to find a common denominator and add fractions with different denominators, students can be prepared for these and other real-life applications.

Least common multiple (LCM)

The least common multiple (LCM) is a fundamental concept in mathematics, particularly in the context of adding fractions with different denominators. It plays a pivotal role in finding a common denominator, which is essential for adding fractions with unlike denominators. Understanding the LCM and its significance in adding fractions helps students develop a deeper understanding of fractions and their operations.

Finding the LCM: The LCM is the smallest positive integer that is divisible by all the denominators of the fractions being added. It is the common denominator that allows us to add the fractions.
Using the LCM to add fractions: Once the LCM is found, each fraction’s numerator and denominator are multiplied by the quotient of the LCM divided by the fraction’s original denominator. This results in equivalent fractions with the LCM as their common denominator, which can then be added.
Real-life applications: Finding the LCM has practical applications beyond adding fractions. It is used in various fields, such as engineering, physics, and computer science, to find the least common period or interval.

In conclusion, the least common multiple (LCM) is an essential concept for adding fractions with different denominators. It provides a systematic approach to finding a common denominator, enabling the addition of fractions with unlike denominators. Understanding the LCM and its applications helps students develop a strong foundation in fraction operations and prepares them for more advanced mathematical concepts.

Cross-multiplication

Cross-multiplication is a fundamental method for finding equivalent fractions with a common denominator. It is a crucial component of adding fractions with different denominators, which is a key skill in mathematics. By understanding the connection between cross-multiplication and adding fractions with different denominators, students can develop a deeper understanding of fractions and their operations.

Cross-multiplication involves multiplying the numerator of one fraction by the denominator of the other fraction, and vice versa. This results in two products that are equal to each other. For example, to find an equivalent fraction for 1/2 with a denominator of 6, we can use cross-multiplication: 1 6 = 6 and 2 3 = 6. Therefore, 1/2 is equivalent to 3/6.

Cross-multiplication is essential for adding fractions with different denominators because it allows us to find a common denominator. Once we have equivalent fractions with a common denominator, we can simply add the numerators and keep the common denominator. For example, to add 1/2 and 1/3, we can first find equivalent fractions with a common denominator: 1/2 = 3/6 and 1/3 = 2/6. Now we can add the fractions: 3/6 + 2/6 = 5/6.

Cross-multiplication is a valuable tool for adding fractions with different denominators. It is a simple and efficient method that can be used to solve a variety of problems. Worksheets that focus on adding fractions with different denominators often include practice problems that require students to use cross-multiplication. By practicing with these problems, students can develop a strong understanding of cross-multiplication and its applications.

In conclusion, cross-multiplication is a fundamental method for finding equivalent fractions with a common denominator. It is an essential component of adding fractions with different denominators, and it is a valuable tool for solving a variety of problems. Worksheets that focus on adding fractions with different denominators can help students develop a strong understanding of cross-multiplication and its applications.

Simplifying fractions

Simplifying fractions is an essential skill in mathematics, especially when adding fractions with different denominators. A fraction is in its simplest form when the numerator and denominator have no common factors other than 1. Simplifying fractions makes it easier to add, subtract, multiply, and divide fractions.

Finding common factors: The first step in simplifying a fraction is to find the common factors of the numerator and denominator. Common factors are numbers that divide evenly into both the numerator and denominator.
Dividing by common factors: Once you have found the common factors, you can divide both the numerator and denominator by those factors. This will reduce the fraction to its simplest form.
Adding fractions with different denominators: When adding fractions with different denominators, it is often necessary to simplify the fractions first. This will make it easier to find a common denominator and add the fractions.

Simplifying fractions is a valuable skill that can be used to solve a variety of problems. Worksheets that focus on adding fractions with different denominators often include practice problems that require students to simplify fractions. By practicing with these problems, students can develop a strong understanding of how to simplify fractions and add fractions with different denominators.

Adding fractions with different denominators

Adding fractions with different denominators is a fundamental skill in mathematics. It is used in a wide variety of applications, from everyday tasks like cooking and measuring ingredients to more complex problems in science and engineering. Worksheets that focus on adding fractions with different denominators can help students develop a strong foundation in this important skill.

The process of adding fractions with different denominators involves finding a common denominator, converting the fractions to equivalent fractions with the common denominator, and then adding the numerators of the equivalent fractions. The common denominator is the least common multiple of the denominators of the fractions being added.

For example, to add the fractions 1/2 and 1/3, we first need to find the least common multiple of 2 and 3. The least common multiple of 2 and 3 is 6, so we convert the fractions 1/2 and 1/3 to equivalent fractions with a denominator of 6: 1/2 = 3/6 and 1/3 = 2/6. Now we can add the numerators of the equivalent fractions: 3/6 + 2/6 = 5/6.

Adding fractions with different denominators can be challenging for some students. However, with practice, students can develop a strong understanding of this important skill. Worksheets that focus on adding fractions with different denominators can provide students with the practice they need to master this skill.

Adding fractions with different denominators is a fundamental skill in mathematics, and worksheets that focus on this topic can be a valuable tool for students. These worksheets provide practice in finding common denominators, converting fractions to equivalent fractions, and adding the fractions together. By working through these problems, students can develop a strong understanding of the process of adding fractions with different denominators.

There are many benefits to using adding fractions with different denominator worksheets. These worksheets can help students to improve their problem-solving skills, their understanding of fractions, and their overall mathematical fluency. Additionally, these worksheets can help students to prepare for standardized tests, which often include questions on adding fractions with different denominators.

The history of adding fractions with different denominator worksheets is long and varied. These worksheets have been used for centuries to teach students about fractions and how to add them. Over time, the worksheets have evolved to include a variety of different problems and activities, all designed to help students learn and practice this important skill.

FAQs on Adding Fractions with Different Denominators

Adding fractions with different denominators can be a challenging concept for students. These FAQs provide answers to some of the most common questions and misconceptions about this topic.

Question 1: What is the first step in adding fractions with different denominators?

Answer: The first step is to find a common denominator. The common denominator is the least common multiple of the denominators of the fractions being added.

Question 2: How do I find the least common multiple of two or more numbers?

Answer: To find the least common multiple of two or more numbers, list the multiples of each number and find the smallest number that is on all of the lists.

Question 3: What if the denominators of the fractions do not have any common factors?

Answer: If the denominators of the fractions do not have any common factors, then the least common multiple is the product of the denominators.

Question 4: Once I have found the common denominator, what do I do next?

Answer: Once you have found the common denominator, you need to convert each fraction to an equivalent fraction with the common denominator. To do this, multiply the numerator and denominator of each fraction by the same number.

Question 5: How do I add the fractions once they have been converted to equivalent fractions?

Answer: To add the fractions once they have been converted to equivalent fractions, simply add the numerators and keep the common denominator.

Question 6: What are some tips for adding fractions with different denominators?

Answer: Here are some tips for adding fractions with different denominators:

Practice regularly.
Understand the concepts of common denominators and equivalent fractions.
Simplify fractions whenever possible.
Check your answers by subtracting the fractions.

Summary: Adding fractions with different denominators can be a challenging concept, but it is an important skill to master. By following these tips and practicing regularly, you can improve your understanding of this topic.

Transition to the next article section: Now that you have a better understanding of adding fractions with different denominators, you can move on to more advanced topics, such as subtracting and multiplying fractions.

Conclusion

Adding fractions with different denominators is a fundamental skill in mathematics. This article has explored the key concepts and steps involved in adding fractions with different denominators, and has provided practice problems to help you master this skill.

Remember, the key to success is practice. The more you practice, the better you will become at adding fractions with different denominators. So keep practicing, and you will soon be a master of this important skill.